Температурна инверсия

Вижте пояснителната страница за други значения на инверсия.
Температурна инверсия (Полша)

Температурна инверсия в метеорологията е явление, при което се наблюдава повишаване на температурата на въздуха с повишаване на надморската височина, вместо нормалното понижаване на температурата във височина. Инверсията на температурите се наблюдава, когато на известна височина, например на 2000 m, си появи „език“ топъл въздух. Това явление се нарича още фронтална инверсия и се дължи на адвекцията на топъл въздух във височина. При други случаи, главна през зимата, в котловините вследствие на радиационно изстиване се натрупва студен въздух, а във височина температурата остава по-висока. Това са т.нар. радиационни инверсии. Такива инверсии се наблюдават най-често в Софийско, Ихтиманско, Трънско, Самоковско, Пернишко, Кюстендилско и други котловинни полета. Много пъти през зимата в София е наблюдавана по-ниска температура, отколкото по оградните възвишения и дори на Черни връх.[1]

Въздействие на замърсяването на въздуха

Поради своята устойчивост с времето се увеличава зоната на натрупване на замърсяване на въздуха, което затруднява хоризонталното диспергиране (разпръскване). Увеличената зона, на височина на която може да бъде, всъщност функционира като „преграда“ или „таван“, така че слоевете над слоя на увеличената зона са на значително метереологично ниво. Поради този факт температурната инверсия има голямо значение по отношение на замърсяването на въздуха.

Вижте също

Външни препратки

Източници

  1. ↑ «Обща физическа география, С., 1977 г.» – Температура на въздуха (Вертикално разпределение на температурата), стр. 62 – 63

Read other articles:

Althaea (mythology)

Character in Greek mythology Althaea or Althea (/ælˈθiːə/; Ancient Greek: Ἀλθαία Althaía healer from ἀλθαίνω althaino, to cure, also a kind of mallow)[1][2] was the queen of Calydon in Greek mythology. Sketch illustration of Althea Family Althaea was the daughter of King Thestius[3] and Eurythemis, and was sister to Leda, Hypermnestra, Iphiclus, Euippus.[4] She was also the wife of Oeneus, king of Calydon, and mother of sons, Meleager, Toxe...

 

Русская народная песня

Эта статья должна быть полностью переписана.На странице обсуждения могут быть пояснения. См. также: Русская песня Митрофан Пятницкий с исполнителями народных песен Ансамбль «Пересек» исполняет народную песню Пение колядок на дореволюционной рождественской открытке Р�...

 

San Gabriel River (Texas)

This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: San Gabriel River Texas – news · newspapers · books · scholar · JSTOR (November 2021) (Learn how and when to remove this template message) San Gabriel RiverRío San GabrielFlood of summer 2007LocationCountryUnited StatesPhysical characteristicsSource  •...

Gusti

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Gusti – berita · surat kabar · buku · cendekiawan · JSTOR Gusti adalah gelar kebangsawanan yang umumnya dimiliki oleh kerajaan-kerajaan di Indonesia yang mendapat pengaruh dari kerajaan-kerajaan (Bahasa ...

 

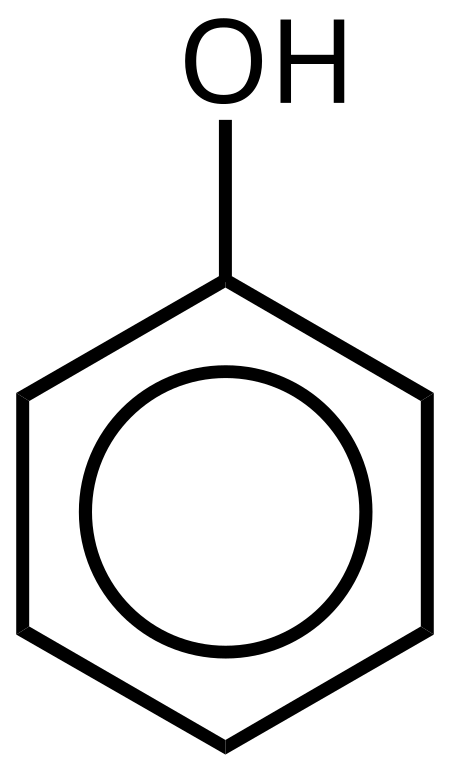
Fenol

Fenol Nama Nama IUPAC Phenol Nama lain Asam karbolat, Benzenol, Asam fenilat, Hidroksibenzena, Asam fenat Penanda Nomor CAS 108-95-2 Y Model 3D (JSmol) Gambar interaktif 3DMet {{{3DMet}}} ChEBI CHEBI:15882 Y ChEMBL ChEMBL14060 Y ChemSpider 971 Y DrugBank DB03255 Y Nomor EC KEGG D06536 Y PubChem CID 996 Nomor RTECS {{{value}}} UNII 339NCG44TV Y CompTox Dashboard (EPA) DTXSID5021124 InChI InChI=1S/C6H6O/c7-6-4-2-1-3-5-6/h1-5,7H YKey: ISWSIDIOOBJBQZ-...

 

List of British representatives in the Trucial States

Trucial States (grey), shown with the Sultanate of Muscat and Oman (red) and the Imamate of Oman (orange). Emirates of the UAE. This is a list of British representatives in the Trucial States from 1939 to 1971. They were responsible for representing British interests in the Trucial States, a British protectorate consisting of seven emirates in the Persian Gulf, which formed the United Arab Emirates (UAE) after the protectorate ended. For British representatives in the UAE since 1971, see: Li...

ロバート・デ・ニーロ

ロバート・デ・ニーロRobert De Niro 2011年のデ・ニーロ生年月日 (1943-08-17) 1943年8月17日（80歳）出生地 アメリカ合衆国・ニューヨーク州ニューヨーク市身長 177 cm職業 俳優、映画監督、映画プロデューサージャンル 映画、テレビドラマ活動期間 1963年 -配偶者 ダイアン・アボット（1976年 - 1988年）グレイス・ハイタワー（1997年 - ）主な作品 『ミーン・ストリート』（1973年）...

 

Frédéric Ier de Bohême

Frédéric Ier de BohêmeLe duc Frédéric de Bohême.FonctionsDuc de Bohêmeseptembre 1182 - 1189Conrad II de BohêmeConrad II de BohêmeDuc de Bohême1178 - juillet 1182Sobeslav II de BohêmeConrad II de BohêmeDuc de Bohême1172-1173Vladislav II de BohêmeSobeslav II de BohêmeTitre de noblesseDuc de BohêmeBiographieNaissance 1142Lieu inconnuDécès 25 mars 1189Lieu inconnuSépulture Cathédrale Saint-Guy de PragueNom dans la langue maternelle BedřichA...

 

Xi Jinping Thought

Set of policies and ideals from Chinese leader Xi Jinping General Secretary of the Chinese Communist Party Xi Jinping for whom the political thought is named. Xi Jinping Thought on Socialismwith Chinese Characteristicsfor a New EraSimplified Chinese习近平新时代中国特色社会主义思想Traditional Chinese習近平新時代中國特色社會主義思想TranscriptionsStandard MandarinHanyu PinyinXí Jìnpíng xīn shídài Zhōngguó tèsè shèhuì zhǔyì sīxiǎngWade–GilesH...

Yuni, Hokkaido

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Yuni, Hokkaido – berita · surat kabar · buku · cendekiawan · JSTOR Yuni 由仁町KotaprajaBalai Kota Yuni BenderaEmblemLokasi Yuni di Hokkaido (Subprefektur Sorachi)YuniLokasi di JepangKoordinat: 43°0�...

 

Patom Highlands

Patom HighlandsПатомское нагорьеGeological Map of the Lena GoldfieldsHighest pointPeakunnamedElevation1,904 m (6,247 ft)Coordinates57°39′08″N 117°47′19″E / 57.65222°N 117.78861°E / 57.65222; 117.78861DimensionsLength500 km (310 mi)Width300 km (190 mi)GeographyPatom HighlandsLocation in Irkutsk Oblast, Russia CountryRussiaFederal subjectIrkutsk Oblast/Zabaykalsky KraiRange coordinates59°N 115°E / &...

 

Wynonna Judd

American country singer (born 1964) Wynonna JuddJudd in 2018BornChristina Claire Ciminella (1964-05-30) May 30, 1964 (age 60)Ashland, Kentucky, U.S.OccupationsSingertelevision personalityYears active1983–presentSpouses Arch Kelley III ​ ​(m. 1996, divorced)​ D. R. Roach ​ ​(m. 2003; div. 2007)​ Scott Cactus Moser ​ ​(m. 2012)​Children2ParentNaomi Judd (moth...

Bodysurfing

Riding a wave without the assistance of any buoyant device Bodysurfer redirects here. For the Australian mini series, see Bodysurfer (miniseries). Thomas VanMelum navigates a glassy right Bodysurfing is the art and sport of riding a wave without the assistance of any buoyant device such as a surfboard or bodyboard. Bodysurfers often equip themselves with a pair of swimfins that aid propulsion and help the bodysurfer catch, ride, and kick out of waves. Some bodysurfers also use a wooden or foa...

 

赤斤蒙古卫

明朝关西八卫 赤斤蒙古卫，明朝关西八卫之一，简称赤斤卫，又作赤金卫。 明朝 明朝永乐二年（1404年）元朝丞相苦术之子塔力尼投降明朝，以其所部在赤斤站设置赤斤蒙古千户所，在今甘肃省玉门市西北赤金堡。永乐八年（1410年）升为赤斤卫，正德年间被吐鲁番汗国所破，当地人内徙肃州的南山，赤斤城空。 清朝 清圣祖康熙五十七年（1718年），恢复赤金卫，清世宗雍正...

 

مركز الدراسات القضائية والتدريب

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (أغسطس 2018) مركز الدراسات القضائية والتدريب شعار مركز الدراسات والتدريب: تفاصيل الوكالة الحكومية البلد مصر  الاسم الكامل مركز الدراسات القضائية والتدريب بهيئة قضاي�...

Integer partition

Decomposition of an integer as a sum of positive integers This article is about partitioning an integer. For grouping elements of a set, see Partition of a set. For the partition calculus of sets, see Infinitary combinatorics. For the problem of partitioning a multiset of integers so that each part has the same sum, see Partition problem. Young diagrams associated to the partitions of the positive integers 1 through 8. They are arranged so that images under the reflection about the main diago...

 

Monopolistic competition

Imperfect competition of differentiated products that are not perfect substitutes Short-run equilibrium of the company under monopolistic competition. The company maximises its profits and produces a quantity where the company's marginal revenue (MR) is equal to its marginal cost (MC). The company is able to collect a price based on the average revenue (AR) curve. The difference between the company's average revenue and average cost, multiplied by the quantity sold (Qs), gives the total profi...

 

Mary Thurman

Mary ThurmanThurman pada 1924LahirMary Christiansen(1895-04-27)27 April 1895Richfield, Utah, Amerika SerikatMeninggal22 Desember 1925(1925-12-22) (umur 30)New York City, Amerika SerikatMakamRichfield City CemeteryPendidikanUniversitas UtahPekerjaanPemeran, peraga busanaTahun aktif1915–1925Suami/istri Victor E. Thurman ​ ​(m. 1915; bercerai 1919)​ Mary Thurman (née. Christiansen, 27 April 1895 – 22 Desember 1925) ada...

Naduvazhi

Location of Kerala in India Naduvazhi ( IAST:nātuvāḻi; lit. 'The ruler of the land') were feudatory Nair princes who ruled over microstates that are now administrative parts of Kerala, India.[1][2] They constituted the aristocratic class of Nairs within the Hindu caste system and were either kings themselves or nobility in the service of the kings of Kerala. Function Prior to the British reorganisation of the area now known as Kerala, it was divided into around ten ...

 

Permis de tuer

Cet article possède des paronymes, voir Licensed to Kill et Plus permis de tuer. Permis de tuer Le logo du film en version originale. Données clés Titre original Licence to Kill Réalisation John Glen Scénario Michael G. WilsonRichard Maibaum Musique Michael Kamen Acteurs principaux Timothy DaltonCarey LowellRobert DaviTalisa SotoBenicio del Toro Sociétés de production EON ProductionsDanjaq Pays de production Royaume-Uni États-Unis Genre espionnage Durée 133 minutes Sortie 1989 Séri...