Tensor product bundle
|
Read other articles:

Hypselodoris apolegma Hypselodoris apolegma di Teluk Bima, Indonesia Klasifikasi ilmiah Kerajaan: Animalia Filum: Mollusca Kelas: Gastropoda Subkelas: Heterobranchia Ordo: Nudibranchia Subordo: Doridina Superfamili: Doridoidea Famili: Chromodorididae Genus: Hypselodoris Spesies: H. apolegma Nama binominal Hypselodoris apolegma(Yonow, 2001)[1] Sinonim Risbecia apolegma Yonow, 2001 Hypselodoris apolegma adalah spesies siput laut dari ordo nudibranch, yaitu moluska gastropoda laut ...

Parish in Louisiana, United States Consolidated city-parish in LouisianaLafayette ParishConsolidated city-parishLafayette Parish CourthouseLocation within the U.S. state of LouisianaLouisiana's location within the U.S.Coordinates: 30°13′N 92°04′W / 30.21°N 92.06°W / 30.21; -92.06Country United StatesState LouisianaFoundedJanuary 17, 1823Named forMarquis de la FayetteSeatLafayetteLargest cityLafayetteGovernment • PresidentMonique Blanco Bou...

Purwokerto beralih ke halaman ini, yang bukan mengenai Purwakarta. Kota PurwokertoIbu kota kabupatenTranskripsi bahasa daerah • Hanacarakaꦥꦹꦂꦮ꧀ꦮꦏꦽꦠ • PegonبوروكيرتوPemandangan Monumen Jenderal Gatot Soebroto di PurwokertoJulukan: Kota SatriaKota MendoanKota PensiunanKota Seribu CurugKota PurwokertoTampilkan peta JawaKota PurwokertoTampilkan peta IndonesiaKoordinat: 7°26′S 109°14′E / 7.433°S 109.233°E ...

Departemen Cerro Largo BenderaLambang kebesaranNegara UruguayIbu kota DepartemenMeloPemerintahan • IntendanSergio Botana • Partai berkuasaPartido NacionalLuas • Total13,648 km2 (5,270 sq mi)Populasi (2011 census) • Total84.698 • Kepadatan6,200/km2 (16,000/sq mi)Demonimarachán, cerrolarguenseZona waktuUTC-3 (UYT)Kode ISO 3166UY-CLSitus webOfficial site The Historic site of the Old Posta del Chuy, ne...

Disambiguazione – Se stai cercando altri significati, vedi Lippe (disambigua). Questa voce o sezione sull'argomento stati scomparsi non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Segui i suggerimenti del progetto di riferimento. Principato di Lippe Principato di Lippe - Localizzazione Dati amministrativiNome completoPrincipato Lippe Nome ufficialeFür...

Dewan Perwakilan RakyatKota Langsa DPRK Langsa2019-2024JenisJenisUnikameral Jangka waktu5 tahunSejarahSesi baru dimulai2 September 2019PimpinanKetuaZulkifli Latief (PA) sejak 30 Desember 2019 Wakil Ketua ISaifullah (Golkar) sejak 28 Oktober 2019 Wakil Ketua IIIr. Joni (Demokrat) sejak 28 Oktober 2019 KomposisiAnggota25Partai & kursi PDI-P (1) NasDem (1) Hanura (3) Demokrat (4) Golkar (4) Gerindra (3) ...

Municipality in Catalonia, SpainDeltebreMunicipality Coat of armsDeltebreLocation in CataloniaShow map of Province of TarragonaDeltebreDeltebre (Catalonia)Show map of CataloniaDeltebreDeltebre (Spain)Show map of SpainCoordinates: 40°43′18″N 0°43′23″E / 40.72167°N 0.72306°E / 40.72167; 0.72306Country SpainCommunity CataloniaProvinceTarragonaComarcaBaix EbreGovernment • MayorLluis Soler Panisello (2015)[1]Area[2] •...

Ghost SweepersSutradaraShin Jung-wonProduserKim Hyeon-seok Lee So-yeong Jang Won-seokSkenarioKwon Seong-hwiCeritaJi Jin-heePemeranKim Su-ro Kang Ye-won Lee Je-hoonPenata musikKim Tae-seongSinematograferSeong Seung-taekPenyuntingKim Chang-juPerusahaanproduksiDasepo ClubSaram EntertainmentDistributorNEWTanggal rilis 03 Oktober 2012 (2012-10-03) Durasi107 minutesNegaraSouth KoreaBahasaKorean Ghost Sweepers (Hangul: 점쟁이들; RR: Jeomjaengyideul; MR:&#...

Голубянки Самец голубянки икар Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ПервичноротыеБез ранга:ЛиняющиеБез ранга:PanarthropodaТип:ЧленистоногиеПодтип:ТрахейнодышащиеНадкласс:ШестиногиеКласс...

ヨハネス12世 第130代 ローマ教皇 教皇就任 955年12月16日教皇離任 964年5月14日先代 アガペトゥス2世次代 レオ8世個人情報出生 937年スポレート公国(中部イタリア)スポレート死去 964年5月14日 教皇領、ローマ原国籍 スポレート公国親 父アルベリーコ2世(スポレート公)、母アルダその他のヨハネステンプレートを表示 ヨハネス12世(Ioannes XII、937年 - 964年5月14日)は、ロ...

College basketball tournament 2014 ACC men's basketball tournament2014 ACC Tournament logoClassificationDivision ISeason2013–14Teams15SiteGreensboro ColiseumGreensboro, North CarolinaChampionsVirginia (2nd title)Winning coachTony Bennett (1st title)MVPJoe Harris (Virginia)TelevisionESPN, ACCNACC men's basketball tournaments← 20132015 → 2013–14 ACC men's basketball standings vte Conf Overall Team W L PCT W L PCT No. 3 Virgini...

American politician (1849–1908) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Oakes Murphy – news · newspapers · books · scholar · JSTOR (July 2022) (Learn how and when to remove this message) Nathan Oakes Murphy10th and 14th Governor of Arizona TerritoryIn officeAugust 1, 1898 – July 1, 1...

Study of the Holocaust Holocaust Studies redirects here. For the academic journal, see Holocaust Studies (journal). Holocaust studies, or sometimes Holocaust research, is a scholarly discipline that encompasses the historical research and study of the Holocaust. Institutions dedicated to Holocaust research investigate the multidisciplinary and interdisciplinary aspects of Holocaust methodology, demography, sociology, and psychology. It also covers the study of Nazi Germany, World War II, Jewi...

此條目需要补充更多来源。 (2016年2月20日)请协助補充多方面可靠来源以改善这篇条目,无法查证的内容可能會因為异议提出而被移除。致使用者:请搜索一下条目的标题(来源搜索:HUNTER×HUNTER角色列表 — 网页、新闻、书籍、学术、图像),以检查网络上是否存在该主题的更多可靠来源(判定指引)。 此条目或其章节有關正在連載或尚未完結的作品。維基百科不是新聞�...

Type of non-standard or temporary employment For the general condition, global perspectives, see Precarity. Precarious work is a term that critics use to describe non-standard or temporary employment that may be poorly paid, insecure, unprotected, and unable to support a household.[1] From this perspective, globalization, the shift from the manufacturing sector to the service sector, and the spread of information technology have created a new economy which demands flexibility in the w...

Property of two varying quantities with a constant ratio For other uses, see Proportionality. This article includes a list of general references, but it lacks sufficient corresponding inline citations. Please help to improve this article by introducing more precise citations. (August 2021) (Learn how and when to remove this message) The variable y is directly proportional to the variable x with proportionality constant ~0.6. The variable y is inversely proportional to the variable x with prop...

「くちぶ」と読む「口部」とは異なります。 この項目には、JIS X 0212-1990、JIS X 0213:2004 で規定されている文字、簡体字、ハングルが含まれています(詳細)。 囗 康熙字典 214 部首 口部 囗部 土部 1 一 丨 丶 丿 乙 亅 2 二 亠 人 儿 入 八 冂 冖 冫 几 凵 刀 力 勹 匕 匚 匸 十 卜 卩 厂 厶 又 3 口 囗 土 士 夂 夊 夕 大 女 子 宀 寸 小 尢 尸 屮 山 巛 工 己 巾 干 幺 广 廴 廾 弋 弓 ...
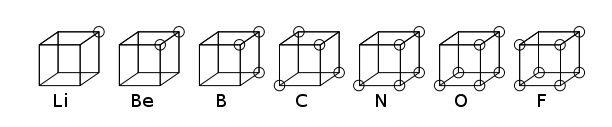
Early atomic model The cubical atom was an early atomic model in which electrons were positioned at the eight corners of a cube in a non-polar atom or molecule. This theory was developed in 1902 by Gilbert N. Lewis and published in 1916 in the article The Atom and the Molecule and used to account for the phenomenon of valency.[1] Lewis' theory was based on Abegg's rule. It was further developed in 1919 by Irving Langmuir as the cubical octet atom.[2] The figure below shows str...

British politician and barrister The Right HonourableThe Lord KenyonPCLord Kenyon, by William Davison (floruit 1843)Lord Chief JusticeIn office4 June 1788 – 11 April 1802MonarchGeorge IIIPreceded byThe Earl of MansfieldSucceeded byThe Lord EllenboroughMaster of the RollsIn office30 March 1784 – 4 June 1788Preceded bySir Thomas SewellSucceeded bySir Richard Arden(later Lord Alvanley)Attorney GeneralIn office26 December 1783 – 31 March 1784Prime MinisterPitt the...

Competitive physical activity using bicycles Bike racing redirects here. Not to be confused with Motorbike racing. Bicycle race redirects here. For the Queen song, see Bicycle Race. For the magazine, see Cycle Sport (magazine). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Cycle sport – news · newspapers · books ...



