Read other articles:

In sociolinguistics, the curvilinear principle states that there is a tendency for linguistic change from below to originate from members of the central classes in a speech community's socioeconomic hierarchy, rather than from the outermost or exterior classes.[1] Overview Graph demonstrating the curvilinear principle, showing that the frequency of use is highest in the interior classes and lowest in the exterior classes. First proposed by William Labov,[2] the curvilinear pri...

Questa voce o sezione sull'argomento citologia non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Segui i suggerimenti del progetto di riferimento. Gli organelli di una cellula vegetale Gli organelli di una cellula animale Gli organuli[1] o organelli[2] sono strutture provviste di membrana presenti all'interno della cellula eucariota che svo...

Not to be confused with KALK. Radio station in Tom Bean, TexasKLAKTom Bean, TexasBroadcast areaNortheast Dallas–Fort Worth metroplexMcKinney/Sherman, Texas & Durant, OklahomaFrequency97.5 MHz (HD Radio)Branding97.5 K-LakeProgrammingLanguage(s)EnglishFormatAdult contemporaryOwnershipOwnerAlpha Media(Alpha Media Licensee LLC Debtor in Possession)Sister stationsKMAD, KMKTHistoryFirst air dateOctober 17, 1983 (at 104.9)Former frequencies104.9 MHz (1983-1987)Call sign meaningK LAKe TexomaTec...

Open source raster graphics editor For other uses, see Gimp (disambiguation). GNU Image Manipulation ProgramGIMP version 2.10Original author(s)Spencer Kimball, Peter MattisDeveloper(s)GIMP Development TeamInitial release2 June 1998; 25 years ago (1998-06-02)Stable release2.10.36[1]  / 7 November 2023Preview release2.99.18[2]  / 21 February 2024 Repositorygitlab.gnome.org/GNOME/gimpWritten inC, C++, Python, SchemeOperating systemLinux, macOS, Windows...

Pour les articles homonymes, voir Long John Silver (homonymie). Cet article est une ébauche concernant une entreprise. Vous pouvez partager vos connaissances en l’améliorant (comment ?). Une page sur une entreprise étant sujette à controverse, n’oubliez pas d’indiquer dans l’article les critères qui le rendent admissible. Long John Silver's Création 1969 Forme juridique LJS Partners Siège social Louisville, Kentucky États-Unis Actionnaires Yum! Brands Activité Rest...

ProSiebenCaractéristiquesCréation 1er janvier 1989Propriétaire ProSiebenSat.1 MediaSlogan « We love to entertain you »Format d'image 576i (SDTV), 720p (HDTV)Langue AllemandPays AllemagneStatut Généraliste nationale privéeSiège social UnterföhringSite web www.prosieben.deDiffusionDiffusion Numérique terrestre, satellite, câble, xDSL et Web.modifier - modifier le code - modifier Wikidata ProSieben est une chaîne de télévision généraliste privée allemande appart...

Voce principale: Paganese Calcio 1926. Unione Sportiva PaganeseStagione 1976-1977 Sport calcio Squadra Paganese Allenatore Gennaro Rambone Presidente Dino Malet Serie C2º posto, girone C Coppa Italia SemiproSemifinale Maggiori presenzeCampionato: Zana (38) Miglior marcatoreCampionato: Albano (5) 1977-1978 Si invita a seguire il modello di voce Questa voce raccoglie le informazioni riguardanti la Unione Sportiva Paganese nelle competizioni ufficiali della stagione 1976-1977. Indice 1 St...

Species of flowering plant Cubeb redirects here. For the West African pepper Piper guineense, see West African pepper. Cubeb Scientific classification Kingdom: Plantae Clade: Tracheophytes Clade: Angiosperms Clade: Magnoliids Order: Piperales Family: Piperaceae Genus: Piper Species: P. cubeba Binomial name Piper cubebaL.f. Piper cubeba, cubeb or tailed pepper is a plant in genus Piper, cultivated for its fruit and essential oil. It is mostly grown in Java and Sumatra, hence sometimes cal...

Chronologies Données clés 1993 1994 1995  1996  1997 1998 1999Décennies :1960 1970 1980  1990  2000 2010 2020Siècles :XVIIIe XIXe  XXe  XXIe XXIIeMillénaires :-Ier Ier  IIe  IIIe Chronologies géographiques Afrique Afrique du Sud, Algérie, Angola, Bénin, Botswana, Burkina Faso, Burundi, Cameroun, Cap-Vert, République centrafricaine, Comores, République du Congo, République démocratique du Congo, Côte d'Ivoire, Djibouti, Égyp...

« Méditerranée » redirige ici. Pour les autres significations, voir Méditerranée (homonymie). La mer Méditerranée est le prototype des mers méditerranéennes. Mer Méditerranée La mer Méditerranée, ses mers secondaires et les zones économiques exclusives. Géographie humaine Pays côtiers Europe Espagne Gibraltar (territoire britannique d'outre-mer) France Monaco Italie Malte Slovénie Croatie Bosnie-Herzégovine Monténégro Albanie GrèceAsie de l'Ouest Turquie Chypr...

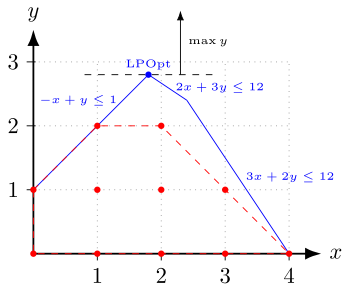
A mathematical optimization problem restricted to integers An integer programming problem is a mathematical optimization or feasibility program in which some or all of the variables are restricted to be integers. In many settings the term refers to integer linear programming (ILP), in which the objective function and the constraints (other than the integer constraints) are linear. Integer programming is NP-complete. In particular, the special case of 0–1 integer linear programming, in which...

Rugby league team season 1908 Eastern Suburbs season1908 NSWRFL season Rank2Play-off resultWon1908 recordWins: 9; draws: 0; losses: 2Points scoredFor: 218; against: 114Team informationCaptainHarry FleggStadiumRoyal Agricultural Society Showground Top scorersTriesHorrie Miller(15)GoalsHerb Brackenreg(17)PointsHorrie Miller(47) Home colours 1909 → The 1908 season was the first in the history of the Eastern Suburbs District Rugby League Football Club (now known a...

Shorinji Kempo (少林寺拳法)FokusCampuranKekerasanKontak penuhNegara asal JepangPenciptaDoshin SoPraktisi terkenalSonny ChibaOrang tuaKung Fu ShaolinOlahraga olimpikTidak Shorinji Kempo (少林寺拳法) adalah salah satu dari seni bela diri yang berasal dari Jepang. Di Indonesia biasa disebut dengan Kempo saja. Shorinji Kempo diciptakan oleh Doshin So[1] (宗 道臣) pada tahun 1947 sebagai sistem pelatihan dan pengembangan diri (行: gyo atau disiplin dalam bahasa jepang).[2...

Koordinat: 6°15′33″S 107°01′13″E / 6.259178°S 107.020154°E / -6.259178; 107.020154 Untuk kegunaan lain, lihat BTC (disambiguasi).Sumber referensi dari artikel ini belum dipastikan dan mungkin isinya tidak benar. Mohon periksa, kembangkan artikel ini, dan tambahkan sumber yang benar pada bagian yang diperlukan. (Pelajari cara dan kapan saatnya untuk menghapus pesan templat ini)Artikel ini perlu dikembangkan agar dapat memenuhi kriteria sebagai entri Wikipedi...

Configuration of mast and rigging for a type of sailboat J-Class sail plan with Bermuda Rig c. 1930 A Bermuda rig, Bermudian rig, or Marconi rig is a configuration of mast and rigging for a type of sailboat and is the typical configuration for most modern sailboats. This configuration was developed in Bermuda in the 17th century; the term Marconi, a reference to the inventor of the radio, Guglielmo Marconi, became associated with this configuration in the early 20th century, because the wires...

Tateyama Cable Car carriage Along the line This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Tateyama Cable Car – news · newspapers · books · scholar · JSTOR (April 2019) (Learn how and when to remove this message)The Tateyama Cable Car (立山ケーブルカー) is a Japanese funicular line of Tateyama Kurobe Kankō (立山黒...

American politician William Abner StanfillUnited States Senatorfrom KentuckyIn officeNovember 19, 1945 – November 5, 1946Appointed bySimeon WillisPreceded byHappy ChandlerSucceeded byJohn S. Cooper Personal detailsBorn(1892-01-16)January 16, 1892Barbourville, KentuckyDiedJune 12, 1971(1971-06-12) (aged 79)Lexington, KentuckyPolitical partyRepublicanAlma materUnion CollegeUniversity of Kentucky William Abner Stanfill (January 16, 1892 – June 12, 1971) was briefly...

World's fair in Porto, Portugal, in 1865 This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: 1865 International Exhibition – news · newspapers · books · scholar · JSTOR (December 2016) (Learn how and when to remove this message) Wood-engraving, published in The Illustrated London News, of the Palácio de Cristal...

2017 film OffendersFilm posterDirected byDejan ZečevićWritten byDjordje MilosavljevicStarringRadovan VujovicRelease date 22 October 2017 (2017-10-22) (Chicago IFF) Running time107 minutesCountrySerbiaLanguageSerbian Offenders (Serbian: Izgrednici) is a 2017 Serbian drama film directed by Dejan Zečević. It was selected as the Serbian entry for the Best Foreign Language Film at the 91st Academy Awards, but it was not nominated.[1][2] Cast Radovan Vujović ...

 Portaal Landen & Volken Staatkundige kaart van de wereld in 2023 Hieronder volgt een lijst van landen van de wereld in 2023. Uitleg Er zijn 196 internationaal erkende onafhankelijke staten. Dat zijn de 193 leden van de Verenigde Naties plus Palestina en Vaticaanstad, die beide een waarnemersstatus hebben bij de VN, en Kosovo.[1] Al deze staten worden door een meerderheid van de landen van de Verenigde Naties erkend als onafhankelijk.[2] Landen die door een kleiner a...