Pasting lemma
|
Read other articles:

Untuk kegunaan lain, lihat Douban (disambiguasi). Douban豆瓣URLdouban.comTipeWeb 2.0, Layanan jejaring sosial, musik daring, basis data film dan bukuPerdagangan ?YaRegistration (en)OpsionalLangueTionghoaBahasa pemrogramanPython PembuatYang Bo (en) Service entry (en)6 Maret 2005; 19 tahun lalu (2005-03-06)Lokasi kantor pusatBeijing NegaraRepublik Rakyat Tiongkok Peringkat Alexa▲ 182, 43 Tiongkok (September 2018[update])[1]KeadaanAktifBlog resmihttps://blog.douban....

2014 greatest hits album by NSYNCThe Essential *NSYNCGreatest hits album by NSYNCReleasedJuly 29, 2014[1]GenrePop, R&BLength1:39:14Label RCA/Jive Legacy ProducerJan Van Der ToornDenniz PopMax MartinKristian LundinDan MuckalaCarl Sturken and Evan RogersVeit RennDavid FosterRami YacoubRichard MarxRobin WileyThe NeptunesBTAllstar GordonNSYNC chronology The Collection(2010) The Essential *NSYNC(2014) Professional ratingsReview scoresSourceRatingAllMusic[2] The Essentia...

Al-Hasa atau al-Ahsa (Arab: الأحساءcode: ar is deprecated al-Aḥsāʾ, al-Ḥasāʾ; Turkish: Lahsacode: tr is deprecated ) adalah wilayah oasis tradisional di Arab Saudi timur yang namanya digunakan untuk Gubernuran Al-Ahsa, yang meliputi Provinsi Timur Arab Saudi. Daerah ini juga sebagai salah satu penagahasil permata - permata yang berharga di jazirah arab. Pranala luar Tourism Portal of Al-Ahsa [pranala nonaktif permanen] Kisahislami.my.id Artikel bertopik geografi atau te...

Sergio Osmeña Presiden Filipina ke-4 Presiden Persemakmuran ke-2Masa jabatan1 Agustus 1944 – 28 Mei 1946Wakil Presidentdk ada PendahuluJosé P. Laurel (de facto)PenggantiManuel RoxasWakil Presiden Filipina ke-1Masa jabatan15 November 1935 – 1 Agustus 1944PresidenManuel L. Quezon PendahuluMariano TríasFrancisco CarreónPenggantiElpidio QuirinoSekretaris Instruksi Umum, Kesehatan, dan Kesejahteraan MasyarakatMasa jabatan1941–1944PresidenManuel L. Quezon PendahuluJorge ...

Jimmy IovineJimmy Iovine bersama Mary J. Blige.PekerjaanProduser MusikTahun aktif1973–sekarangSitus webJimmy Iovine James Jimmy Iovine (lahir 11 Maret 1953) adalah seorang produser musik, pengusaha dan ketua Interscope-Geffen-A&M. Referensi Pranala luar Interview with Artists House Music Diarsipkan 2010-12-23 di Wayback Machine. Interscope Records Biography at www.fleetwoodmac.net Persondata Nama Iovine, Jimmy Nama alternatif Deskripsi singkat Tanggal lahir March 11, 1953 Tempat l...

Radio station in Gallup, New MexicoKFMQGallup, New MexicoFrequency106.1 MHzBrandingRock 106.1ProgrammingFormatMainstream rockAffiliationsPremiere NetworksOwnershipOwneriHeartMedia, Inc.(iHM Licenses, LLC)Sister stationsKFXR-FM, KGLX, KXTCHistoryFirst air date1994; 30 years ago (1994)Technical information[1]Licensing authorityFCCFacility ID40806ClassC1ERP100,000 wattsHAAT57 meters (187 ft)Transmitter coordinates35°29′39″N 108°44′32″W / 3...

Cet article est une ébauche concernant une localité slovaque. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Podbrezová Administration Pays Slovaquie Région Banská Bystrica District Brezno Statut Village Starosta (maire) Mandat Ladislav Kardhordó (Indépendant) mandat : 2018-2022 Code postal 976 81 Plaqueminéralogique BR Code LAU 2 508853 Démographie Population 3 860 hab. (31 déc. 2018) ...

British recipient of the Victoria Cross (1873–1952) Samuel VickeryBorn(1873-02-06)6 February 1873Wambrook, SomersetDied20 June 1952(1952-06-20) (aged 79)Cardiff, WalesAllegiance United KingdomService/branch British ArmyRankCorporalUnitThe Dorsetshire RegimentBattles/wars Tirah Campaign Second Boer War World War I AwardsVictoria Cross Samuel Vickery VC (6 February 1873 – 20 June 1952) was a British recipient of the Victoria Cross, the highest and most prestigious award f...

Greek island in the Ionian Sea For other uses, see Corfu (disambiguation). Corcyra redirects here. For other uses, see Corcyra (disambiguation). CorfuNative name: ΚέρκυραNickname: Το νησί των Φαιάκων (The island of Faiakes)GeographyCoordinates39°36′N 19°52′E / 39.60°N 19.87°E / 39.60; 19.87Area610.9 km2 (235.9 sq mi)Highest elevation906 m (2972 ft)Administration GreeceAdministrative regionIonian IslandsR...

Disambiguazione – Taiwanesi rimanda qui. Se stai cercando i popoli indigeni dell'isola, vedi Aborigeni di Taiwan. Questa voce o sezione sull'argomento Taiwan non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Taiwan (dettagli) (dettagli) Taiwan - LocalizzazioneIn verde scuro i territori controllati da Taiwan, in verde chiaro i territori rivendicat...

ХристианствоБиблия Ветхий Завет Новый Завет Евангелие Десять заповедей Нагорная проповедь Апокрифы Бог, Троица Бог Отец Иисус Христос Святой Дух История христианства Апостолы Хронология христианства Раннее христианство Гностическое христианство Вселенские соборы Н...

Суперсемейка 2англ. Incredibles 2 Жанры приключениябоевиккомедиясемейный Техника анимации компьютерная Режиссёр Брэд Бёрд Автор сценария Брэд Бёрд Роли озвучивали Холли Хантер Крэйг Т. Нельсон Сара Вауэлл Хак Милнер Сэмюэл Л. Джексон Брэд Бёрд Джон Ратценбергер Боб Оденк�...

此條目可参照英語維基百科相應條目来扩充。 (2021年5月6日)若您熟悉来源语言和主题,请协助参考外语维基百科扩充条目。请勿直接提交机械翻译,也不要翻译不可靠、低品质内容。依版权协议,译文需在编辑摘要注明来源,或于讨论页顶部标记{{Translated page}}标签。 约翰斯顿环礁Kalama Atoll 美國本土外小島嶼 Johnston Atoll 旗幟颂歌:《星條旗》The Star-Spangled Banner約翰斯頓環礁�...
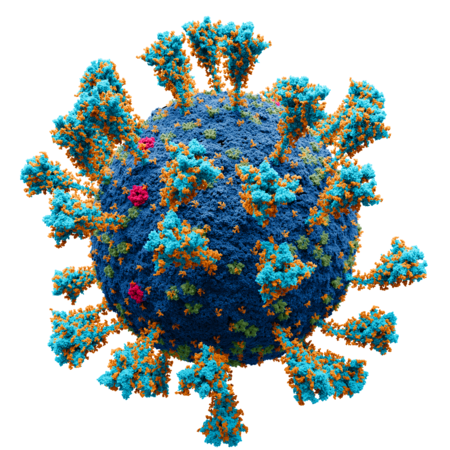
جائحة فيروس كورونا في هندوراس 2020 المرض مرض فيروس كورونا 2019 السلالة فيروس كورونا المرتبط بالمتلازمة التنفسية الحادة الشديدة النوع 2 التواريخ 10 مارس 2020(4 سنوات، و2 شهور، و1 أسبوع، و4 أيام) المنشأ الصين المكان هندوراس الوفيات 11,111 (9 مارس 2023) الحالات المؤك...

Overview of ethnic groups in the United Kingdom This article is about ethnic groups in the United Kingdom regardless of birthplace. For foreign-born groups in the United Kingdom, see Foreign-born population of the United Kingdom. Part of a series on theCulture of the United Kingdom History Georgian period Napoleonic Wars Regency period Victorian period British Empire Edwardian period First World War Interwar period Second World War Post-war period (social history) Brexit PeopleHistoric people...

Free trade agreement signed on 2020 See also: Canada–United Kingdom Free Trade Agreement UK - Canada Trade Continuity Agreement (TCA)United Kingdom - Canada Trade Continuity Agreement (TCA) United Kingdom (UK) CanadaTypeFree trade agreementContextTrade continuity agreement between Canada and the United KingdomSigned8 December 2020Sealed21 November 2020Effective1 January 2021Negotiators Mary Ng Liz Truss Parties Canada United Kingdom LanguagesEnglishFrench The Canada–...

Al-GhadirPermukimanAl-GhadirLocation in the Kingdom of Saudi ArabiaKoordinat: 24°38′N 46°43′E / 24.633°N 46.717°E / 24.633; 46.717Koordinat: 24°38′N 46°43′E / 24.633°N 46.717°E / 24.633; 46.717Negara Arab SaudiPemerintahan • Gubernur Pangeran RiyadhFaisal bin Bandar Al Saud • Wali kotaIbraheem Mohammed Al-SultanKetinggian612 m (2,008 ft)Zona waktuUTC+3 (AST) • Musim panas (DST)UT...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (أغسطس 2017) وفيما يلي قائمة بأعضاء حركة فتح وهو حزب سياسي فلسطيني رئيسي وميليشيا تأسست في الفترة ما بين 1958 و1959. تشمل القائمة القادة والمسلحين والمحافظين ورؤساء البلديات...

Clifford William King SadlierLieutenant Clifford Sadlier in 1918Nickname(s)BillBorn1892 (1892)Camberwell, VictoriaDied28 April 1964 (1964-04-29) (aged 71)Busselton, Western AustraliaAllegianceAustraliaService/branchAustralian Imperial ForceYears of service1915–19RankLieutenantUnit51st BattalionBattles/warsFirst World War Western Front Second Battle of Villers-Bretonneux AwardsVictoria Cross Clifford William King Sadlier, VC (1892 – 28 April 1964) was an Australian rec...
Philosophical concept For other uses, see Impermanence (disambiguation). This article's factual accuracy is disputed. Relevant discussion may be found on the talk page. Please help to ensure that disputed statements are reliably sourced. (April 2022) (Learn how and when to remove this message) A Buddhist painting displaying Impermanence Impermanence, also known as the philosophical problem of change, is a philosophical concept addressed in a variety of religions and philosophies. In Eastern p...























