Parental responsibility (criminal)

In Canada and the United States, the term parental responsibility refers to the potential or actual liability that may be incurred by parents for the behavior of their children.

In Canada law

Parental responsibility legislation has been enacted in three Canadian provinces: Manitoba (1997), Ontario (2000), and British Columbia (2001).

Under the Parental Responsibility Act, 2000, a "child" is anyone under the age of 18 years, and "parent" means:

  • either the biological, adoptive, or legal guardian parent of the child,
  • or the person who has lawful custody of, or a right of access to, the child.

Small claims court may order such parents to compensate those suffering any loss or damage intentionally caused by their child, unless the parent was exercising reasonable supervision over the child at the time the child engaged in the activity that caused the loss or damage and made reasonable efforts to prevent or discourage the child from engaging in the kind of activity that resulted in the loss or damage.[1]

In U.S. law

There is a political movement for greater parental accountability, following of a number of highly publicized violent crimes committed by children. While all U.S. states allow parents to be sued for the various actions of their children, the idea of criminal legislation to enable the prosecution of adults for “neglectful” parenting is relatively new. For example, a number of states have enacted or proposed laws that will:

  • automatically hold parents financially responsible for all expenses associated with a second false bomb threat or 911 call made by a child;
  • impose a prison term of up to 18 months and order payment of restitution to any victims if the child commits a serious crime;
  • imposes a fine and/or a prison term if a child uses a gun owned by the parent to commit a crime;
  • fine and/or imprison parents whose children fail to attend school or skip school more than 10 times in a year.

Such legislation is constitutionally problematic in that it is often both broad and vague, and may very well infringe upon the privacy of the family. The sponsors of these laws argue that parental delinquency is usually the cause of the juvenile delinquency, but opponents argue that there is little evidence to support the claim that youth crime is caused by “bad parenting” and that there are many other social, cultural and economic causes that should be addressed. Opponents argue that the increased work hours and multiple jobs that a growing number of parents must take to provide for their families' basic needs decreases their ability to supervise their child(ren), thus creating an injustice with regard to the parents' culpability in the child's action. In 1988, California enacted the Street Terrorism and Prevention Act, which provides for prison sentences of up to one year and fines up to $2,500 for parents who “grossly” or “culpably” deviate from the “normal” standards of supervision. The Supreme Court of California later upheld the constitutionality of this act.[2]

References

  1. ^ "E-Laws". 24 July 2014.
  2. ^ Brank, Eve M.; Weisz, Victoria (2004). "Paying for the crimes of their children: Public support of parental responsibility". Journal of Criminal Justice. 32 (5). Science Direct: 465–475. doi:10.1016/j.jcrimjus.2004.06.010.

Read other articles:

B'z The Best XXV 1999–2012

2013 compilation album by B'zB'z The Best XXV 1999–2012Compilation album by B'zReleasedJune 12, 2013GenrePop rock, hard rockLabelVermillion RecordsProducerTak MatsumotoB'z chronology B'z The Best Ultra Treasure(2008) B'z The Best XXV 1999–2012(2013) B'z The Best XXV 1999–2012 is a compilation album by Japanese hard rock duo B'z. It was released on June 12, 2013, simultaneously with B'z The Best XXV 1988–1998, and it is part of their 25th anniversary celebration. It reached #2 ...

 

 

Agama di Iran

Agama di Iran (census 2011)[1]   Syiah (94.1%)  Sunni (5.3%)  Lain lain (0.6%) Iran merupakan sebuah negara beragama. Menurut badan statistik Iran, hanya empat agama saja yang diakui yaitu agama Islam, Kristen, Yahudi dan Majusi. Penganut agama Islam merupakan kumpulan terbesar di negara ini. Sebanyak 99 persen penduduk Iran beragama Islam. Penganut minoritas lain ialah penganut agama Hindu, Sikh, Bahai, Mandean dan Yarsan.[2] Sebuah studi Mei 20...

 

 

Affair

AffairSutradaraNayato Fio NualaProduserChand Parwez ServiaDitulis olehViva WestiPemeranSigi WimalaGarneta HaruniDimas AdityaMonique HenryRozie MahallyPenata musikEka FirdausSinematograferNayato Fio NualaFreddy LinggaPenyuntingTiara Puspa RaniPerusahaanproduksiStarvisionIndie PictureDistributorStarvisionTanggal rilis11 Maret 2010Durasi88 MenitNegara Indonesia Affair adalah film thriller dari Indonesia yang dirilis pada tanggal 11 Maret 2010 dan disutradarai oleh Nayato Fio Nuala. Plot Di...

Nottingham Forest F.C.

Nottingham ForestNama lengkapNottingham Forest YesJulukanForest, The Reds, NFFC, The Tricky Trees,[1] '[2]Berdiri1865StadionCity GroundWest BridgfordNottingham(Kapasitas: 30,602[3])Pemilik Evangelos MarinakisKetua Nicholas Randall QC[4]Manajer Nuno Espírito SantoLigaLiga Utama Inggris2022–2023Liga Utama Inggris, ke-16 dari 20Situs webSitus web resmi klub Kostum kandang Kostum tandang Kostum ketiga Musim ini Nottingham Forest Football Club adalah nama kl...

 

 

العلاقات الإكوادورية المالية

العلاقات الإكوادورية المالية الإكوادور مالي   الإكوادور   مالي تعديل مصدري - تعديل   العلاقات الإكوادورية المالية هي العلاقات الثنائية التي تجمع بين الإكوادور ومالي.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية للدولتين: وجه المقا...

 

 

Karakara berjambul

Karakara Utara (Caracara cheriway) di Kosta Rika Karakara Berjambul adalah nama Falconiformes falconid. Karakara Berjambul terdiri dari: Karakara Utara, Caracara cheriway Karakara Selatan, Caracara plancus Karakara Guadalupe, Caracara lutosa Artikel bertopik hewan ini adalah sebuah rintisan. Anda dapat membantu Wikipedia dengan mengembangkannya.lbs

Banten II (electoral district)

Banten IIElectoral district constituencyfor the People's Representative CouncilDistrict List Regencies : Serang Regency Cities : Serang Cilegon ProvinceBantenCurrent constituencySeats6Member(s)  Ichsan Soelistio (PDI-P)  Jazuli Juwaeni (PKS)  Nuraeni (Democratic Party)  Desmond Junaidi Mahesa (Gerindra)  Haerul Jaman (Golkar)  Yandri Susanto (PAN) Banten II is an electoral district in Indonesia which encompasses the cities of Serang and Cilegon, along w...

 

 

Tiridate Ier d'Arménie

Vous lisez un « bon article » labellisé en 2008. Pour les articles homonymes, voir Titidate Ier. Tiridate Ier d'Arménie Statue de Tiridate Ier d’Arménie par André, dans les jardins du Château de Versailles (rampe nord). Titre Roi d’Arménie 53 – 53(moins d’un an) Prédécesseur Rhadamiste Successeur Rhadamiste 54 – 58(4 ans) Prédécesseur Rhadamiste Successeur Tigrane VI 63 – 72(9 ans) Prédécesseur Tigrane VI Successeur Sanatrocès Ier (?) Biographie...

 

 

Stasiun Manggarai

Stasiun Manggarai A01B09C13 Tampak depan perspektif Stasiun Manggarai sisi timur dengan teks bertuliskan Stasiun Sentral Manggarai pada bangunan lama, Maret 2024LokasiJalan Manggarai Utara 1Manggarai, Tebet, Jakarta Selatan, 12850IndonesiaKoordinat6°12′28″S 106°51′10″E / 6.20778°S 106.85278°E / -6.20778; 106.85278Koordinat: 6°12′28″S 106°51′10″E / 6.20778°S 106.85278°E / -6.20778; 106.85278Ketinggian+13 mOperator KAI Comm...

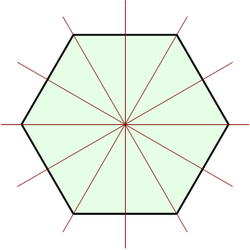
Group representation

Group homomorphism into the general linear group over a vector space Not to be confused with Presentation of a group. A representation of a group acts on an object. A simple example is how the symmetries of a regular polygon, consisting of reflections and rotations, transform the polygon. In the mathematical field of representation theory, group representations describe abstract groups in terms of bijective linear transformations of a vector space to itself (i.e. vector space automorphisms); ...

 

 

برج النمر

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (فبراير 2016) برج النمر برج النمر هو أحد الأبراج الصينية، وينافس برج الأسد في الأبراج الغربية. تقول الأسطورة الصينية ب�...

 

 

Earl Hogan

American politician This article relies largely or entirely on a single source. Relevant discussion may be found on the talk page. Please help improve this article by introducing citations to additional sources.Find sources: Earl Hogan – news · newspapers · books · scholar · JSTOR (February 2017) Earl Lee HoganFrom 1959's Pocket Congressional Directory of the 86th CongressMember of the U.S. House of Representativesfrom Indiana's 9th distric...

Bugesera invasion

1963 Inyenzi rebels attacks and massacres in Rwanda For other uses, see Bloody Christmas. Bugesera invasionDate21–27 December 1963LocationRwandaResult Rwandan government victory Anti-Tutsi massacres in RwandaBelligerents InyenziSupported by: China  RwandaSupported by: BelgiumCommanders and leaders François RukebaKigeli V (disputed) Grégoire KayibandaJuvénal Habyarimana (disputed)Units involved Armée Royale Rwandaise Garde Nationale RwandaiseStrength Hundreds of ...

 

 

Ernest L. Ransome

The 15-story Ingalls Building in Cincinnati, Ohio became the world's first reinforced concrete skyscraper in 1903 Ernest Leslie Ransome (1844–1917[1]) was an English-born engineer, architect, and early innovator in reinforced concrete building techniques. Ransome devised the most sophisticated concrete structures in the United States at the time. Ernest was the son of Frederick Ransome, who had patented a process for producing artificial stone in 1844. Ernest was apprenticed to his...

 

 

Condino

Condino abolished municipality in Italy Condino (it) Tempat Negara berdaulatItaliaDaerah otonom dengan status istimewaTrentino-Tirol SelatanProvinsi di ItaliaTrentinoKomune di ItaliaBorgo Chiese NegaraItalia Ibu kotaCondino GeografiLuas wilayah33,74 km² [convert: unit tak dikenal]Ketinggian444 m Berbatasan denganBagolino Breno Brione Cimego Daone Ledro Storo Castel Condino SejarahSanto pelindungMaria Diangkat ke Surga Informasi tambahanKode pos38083 Zona waktuUTC+1 UTC+2 Kode telepon046...

Yuma Suzuki

Yuma Suzuki oleh Mehdi Bolourian 2018Informasi pribadiNama lengkap Yuma SuzukiTanggal lahir 26 April 1996 (umur 28)Tempat lahir Prefektur Chiba, JepangPosisi bermain PenyerangKarier senior*Tahun Tim Tampil (Gol)2015– Kashima Antlers * Penampilan dan gol di klub senior hanya dihitung dari liga domestik Yuma Suzuki (lahir 26 April 1996) adalah pemain sepak bola asal Jepang. Karier Yuma Suzuki pernah bermain untuk Kashima Antlers. Pranala luar (Jepang) Profil dan statistik di situs web r...

 

 

بطولة العالم للدراجات على المضمار 1989

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (ديسمبر 2018) بطولة العالم للدراجات على المضمار 1989 التفاصيل التاريخ 1989 الموقع  فرنسا (ليون) نوع السباق سباق الدراجات...

 

 

This Girl's in Love with You

1970 studio album by Aretha Franklin This article is about the album by Aretha Franklin. For the song by Burt Bacharach and Hal David sometimes covered with this title, see This Girl's in Love with You (song). This Girl's in Love with YouStudio album by Aretha FranklinReleasedJanuary 15, 1970[1]RecordedJanuary 8–9, 1969[2]October 3, 1969[3]December 1969[4]GenreSoul, R&BLength35:45LabelAtlanticProducerJerry Wexler, Tom Dowd & Arif MardinAretha Fran...

Random matrix

Matrix-valued random variable In probability theory and mathematical physics, a random matrix is a matrix-valued random variable—that is, a matrix in which some or all of its entries are sampled randomly from a probability distribution. Random matrix theory (RMT) is the study of properties of random matrices, often as they become large. RMT provides techniques like mean-field theory, diagrammatic methods, the cavity method, or the replica method to compute quantities like traces, spectr...

 

 

Ministère de l'Écologie (France)

Pour les articles homonymes, voir Ministère de l'Écologie. Ministère de la Transition écologique, de l’énergie, du climat et de la prévention des risques (appellation depuis 2024)Logo en septembre 2024.exposition sur les sites classés dans le salon bleu, à l'Hôtel de Roquelaure.HistoireFondation 1971 : ministère de la Protection de la nature et de l'environnement1978 : ministère de l'Environnement et du Cadre de vie1981 : ministère de l'Environnement1997 : m...