Idealized greenhouse model
|
Read other articles:

Huruf KirilCha Khakasia Alfabet KirilHuruf SlaviaАА́А̀А̂А̄ӒБВГҐДЂЃЕЕ́ÈЕ̂ЁЄЖЗЗ́ЅИИ́ЍИ̂ЙІЇЈКЛЉМНЊОŌПРСС́ТЋЌУУ́ У̀У̂ӮЎФХЦЧЏШЩЪЫЬЭЮЯHuruf non-SlaviaӐА̊А̃Ӓ̄ӔӘӘ́Ә̃ӚВ̌ҒГ̑Г̣Г̌ҔӺҒ̌ӶД̌Д̣Д̆ӖЕ̄Е̃Ё̄Є̈ӁҖӜҘӞЗ̌З̱З̣ԐԐ̈ӠӢИ̃ҊӤҚӃҠҞҜК̣ԚӅԮԒӍӉҢԨӇҤО́О̀О̆О̂О̃ӦӦ̄ӨӨ̄Ө́Ө̆ӪҨԤР̌ҎҪС̣С̱Т̌Т̣ҬУ̃Ӱ Ӱ́Ӱ̄ӲҮҮ́ҰХ̣Х̱Х̮Х̑�...

Psychedelic drug Allylescaline Names Preferred IUPAC name 2-{3,5-Dimethoxy-4-[(prop-2-en-1-yl)oxy]phenyl}ethan-1-amine Other names 2-[4-(Allyloxy)-3,5-dimethoxyphenyl]ethan-1-amine2-[4-(Allyloxy)-3,5-dimethoxyphenyl]ethanamine Identifiers CAS Number 39201-75-7 Y 3D model (JSmol) Interactive image ChEMBL ChEMBL126803 N ChemSpider 21106254 N PubChem CID 4471946971669102 hydrochloride UNII J39IWS08EN Y CompTox Dashboard (EPA) DTXSID80660348 InChI InChI=1S/C13H19NO3/c1-4-...

North, Central America and Caribbean Volleyball ConfederationOlahragaBola voli Voli pantaiYurisdiksiAmerika Tengah dan UtaraJumlah anggota42 negaraSingkatanNORCECABerdiri1968AfiliasiFIVBKantor pusatSanto Domingo,Republik DominikaPresiden Cristóbal Marte HoffizSitus web resmiwww.norceca.org North, Central America and Caribbean Volleyball Confederation (NORCECA) (Indonesia: Konfederasi Bola Voli Amerika Utara dan Tengah) adalah induk organisasi kontinental untuk olahraga bola voli di Amerika U...

Men's Greco-Roman light heavyweightat the Games of the X OlympiadVenueGrand Olympic AuditoriumDates5–7 AugustCompetitors3 from 3 nationsMedalists Rudolf Svensson Sweden Onni Pellinen Finland Mario Gruppioni Italy← 19281936 → Wrestling at the1932 Summer OlympicsGreco-RomanBantammenFeathermenLightmenWeltermenMiddlemenLight heavymenHeavymenFreestyleBantammenFeathermenLightmenWeltermenMiddlemenLight heavymenHeavymenvte The men's Greco-Roman feat...

Indian scientist and conservationist A major contributor to this article appears to have a close connection with its subject. It may require cleanup to comply with Wikipedia's content policies, particularly neutral point of view. Please discuss further on the talk page. (December 2022) (Learn how and when to remove this template message) Yadvendradev Vikramsinh JhalaBorn (1962-02-27) February 27, 1962 (age 62)Mumbai, Maharashtra, IndiaNationalityIndianAlma materUniversity of Mumbai ...

Places in the capital city of Wales This is a listing of places in Cardiff, county and capital city of Wales. Administrative divisions Electoral wards This is a list of electoral wards covered by Cardiff Council: Adamsdown Butetown Caerau Canton Cathays Creigiau & St. Fagans Cyncoed Ely Fairwater Gabalfa Grangetown Heath Llandaff North Llanishen Lisvane & Thornhill Llandaff Llanrumney Pentwyn Pentyrch Penylan Plasnewydd Pontprennau & Old St. Mellons Radyr & Morganstown Rhiwbin...

Conference Held in Moscow This article's lead section may be too short to adequately summarize the key points. Please consider expanding the lead to provide an accessible overview of all important aspects of the article. (January 2023) The Fourth Moscow Conference,[1] also known as the Tolstoy Conference[2] for its code name Tolstoy,[3] was a meeting in Moscow between Winston Churchill and Joseph Stalin from 9 October to 19 October 1944. Procedures Main article: Percen...

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: SMA Negeri 2 Purworejo – berita · surat kabar · buku · cendekiawan · JSTOR SMA Negeri 2 Purworejo atau yang lebih dikenal dengan SMANDA adalah salah satu sekolah favorit di Kabupaten Purworejo, Jawa Teng...

Pour les articles homonymes, voir La Relève. Cet article est une ébauche concernant un film américain. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les conventions filmographiques. La Relève Données clés Titre québécois La Recrue Titre original The Rookie Réalisation Clint Eastwood Scénario Scott SpiegelBoaz Yakin Musique Lennie Niehaus Acteurs principaux Clint EastwoodCharlie SheenRaúl JuliáSonia Braga Sociétés de production Malpaso Producti...

Women's National Basketball Association 2000Dettagli della competizioneSport Pallacanestro OrganizzatoreWNBA Periodo29 maggio 2000 —26 agosto 2000 Data2000 Squadre16 (in 2 gironi) VerdettiTitolo East N.Y. Liberty Titolo West Houston Comets Campione Houston Comets(4º titolo) MVPSheryl Swoopes Miglior allenatoreMichael Cooper Miglior marcatoreSheryl Swoopes MVP delle finaliCynthia Cooper Cronologia della competizioneed. successiva → ← ...
周處除三害The Pig, The Snake and The Pigeon正式版海報基本资料导演黃精甫监制李烈黃江豐動作指導洪昰顥编剧黃精甫主演阮經天袁富華陳以文王淨李李仁謝瓊煖配乐盧律銘林孝親林思妤保卜摄影王金城剪辑黃精甫林雍益制片商一種態度電影股份有限公司片长134分鐘产地 臺灣语言國語粵語台語上映及发行上映日期 2023年10月6日 (2023-10-06)(台灣) 2023年11月2日 (2023-11-02)(香�...

Halaman ini berisi artikel tentang misionaris Kristen India. Untuk pemilik tanah dan politisi India, lihat Sundar Singh Majithia. Sadhu Sundar SinghLahir(1889-09-03)3 September 1889Ludhiana, IndiaMeninggaltidak diketahui, namun diyakini oleh beberapa orang hal tersebut terjadi pada tahun 1929HimalayaPendidikanKolese Anglikan, LahoreGelarSadhu Sadhu Sundar Singh (3 September 1889, Negara Patiala, India) adalah seorang misionaris Kristen India. Kehidupan awal Pada 3 September 1889, di sebelah U...

It B1A4Album mini karya B1A4Dirilis22 September 2011 (2011-09-22)Direkam2011GenreK-pop, Dance-popBahasaKoreanLabelWM EntertainmentKronologi B1A4 Let's Fly(2011)Let's Fly2011 it B1A4(2011) IGNITION(2012)IGNITION2012 Singel dalam album It B1A4 Beautiful TargetDirilis: 15 September 2011 (2011-09-15) My LoveDirilis: 18 November 2011 (2011-11-18) It B1A4 adalah album mini kedua oleh boyband Korea Selatan, B1A4 dan dirilis oleh WM Entertainment pada 16 September 2011. Tracklistin...
It has been suggested that Higher education be merged into this article. (Discuss) Proposed since October 2023. Advanced level of education, usually for adults The examples and perspective in this article may not represent a worldwide view of the subject. You may improve this article, discuss the issue on the talk page, or create a new article, as appropriate. (April 2018) (Learn how and when to remove this message) Students attend a linear algebra lecture at a tertiary institution: Helsinki ...

Evening or entire day before Christmas Day For other uses, see Christmas Eve (disambiguation). Christmas night redirects here. For the album, see Christmas Night. Christmas EveChristmas Eve, an 1878 painting by J. Hoover & SonAlso calledVigil of the NativityChristmas EveningChristmas VigilDay before ChristmasNight before ChristmasObserved byChristiansMany non-Christians[1]TypeChristian, culturalSignificanceDay or evening preceding the traditional birthday of JesusObservancesG...

Medical term Medical conditionLower respiratory tract infectionDepiction of a person with LRTISpecialtyPulmonology Frequency291 million (2015)[1]Deaths2.74 million (2015)[2] Lower respiratory tract infection (LRTI) is a term often used as a synonym for pneumonia but can also be applied to other types of infection including lung abscess and acute bronchitis. Symptoms include shortness of breath, weakness, fever, coughing and fatigue.[3] A routine chest X-ray is not...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (مارس 2016) لنكسمعلومات عامةالتصنيف لغة برمجة ظهرت في 1984 التطويرالمطور جامعة ويسكونسن-ماديسون الإصدار الأول 1984 تعديل ...

The Romani ethnic group in Portugal Ethnic group Portuguese Romanis1892 description of a group of Romani from the AlentejoTotal populationest. 30,000 – 50,000Regions with significant populationsLisbon, Setúbal, Alentejo and AlgarveLanguagesPortuguese, CalóReligionRoman Catholicism Part of a series onRomani people Archaeology Cuisine Culture Dance Dress Folklore History Language Media Music Names People Religion Settlements Romani people by sub-group Arlije Bergitka Roma Burgenland Roma Bo...

Ice hockey team in Södertälje, SwedenSödertälje SKCitySödertälje, SwedenLeagueHockeyAllsvenskanFounded22 February 1902Home arenaScaniarinkenColorsNavy, white, yellow General managerRobert AnderssonHead coachMagnus BogrenCaptainChristoffer LiljewallWebsitesodertaljesk.seFranchise history1902-presentSödertälje SportklubbChampionshipsPlayoff championships1925Le Mat Trophy1931, 1941, 1944, 1953, 1956, 1985 Södertälje Sportklubb, also known as Södertälje SK and...

Voce principale: Prima Categoria 1964-1965. Prima Categoria Marche 1964-1965 Competizione Prima Categoria Sport Calcio Edizione 6ª Organizzatore FIGC - LNDComitato Regionale Marchigiano Luogo Italia Partecipanti 28 Cronologia della competizione 1963-1964 1965-1966 Manuale Il campionato di calcio di Prima Categoria 1964-1965 è stato il V livello del campionato italiano. A carattere regionale, fu il sesto campionato dilettantistico con questo nome dopo la riforma voluta da Zauli del 19...



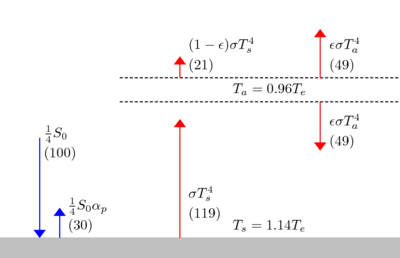







![{\displaystyle T_{s}=\left[{\frac {S_{0}(1-\alpha _{p})}{4\sigma }}{\frac {1}{1-{\epsilon \over 2}}}\right]^{\frac {1}{4}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/95232bef370ba744115539a14dfb600559feed48)
![{\displaystyle T_{e}\equiv \left[{\frac {{\frac {1}{4}}S_{0}(1-\alpha _{p})}{\sigma }}\right]^{\frac {1}{4}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/fa796120e6b61fee258c6326a85816634bf3b45d)
![{\displaystyle T_{s}=T_{e}\left[{\frac {1}{1-{\epsilon \over 2}}}\right]^{\frac {1}{4}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/aa4c3f30f5b7b229c563d4e300c898bfeae68bc8)







