Generalized Lotka–Volterra equation
|
Read other articles:

Patung dada perunggu Johnson karya Jacob Epstein, 1942 Hewlett Johnson (25 Januari 1874 – 22 Oktober 1966) adalah seorang pendeta Inggris dari Gereja Inggris. Ia menjadi Dekan Manchester dan kemudian Dekan Canterbury, dimana ia dijuluki Dekan Merah dari Canterbury karena dukungannya terhadap Uni Soviet dan sekutu-sekutunya. Pranala luar Hewlett Johnson archive at marxists.org Kliping surat kabar tentang Hewlett Johnson di Arsip Pers Abad ke-20 dari Perpustakaan Ekonomi Nasiona...

Bilderberg Hotel Wientjes, Zwolle Bilderberg adalah jaringan hotel dan restoran yang berdiri di Belanda. Saat ini Bilderberg mengoperasikan 20 hotel di Belanda. Namanya menjadi asal usul nama Bilderberg Group yang mengadakan pertemuan pertamanya di Bilderberg Hotel di Oosterbeek. Hotel Hotel-hotel ternama milik jaringan ini adalah: Bilderberg Hotel, Oosterbeek Doorwerth Castle, Doorwerth Restoran ternama milik jaringan ini adalah: De Kromme Dissel, Heelsum De Kersentuin, di Bilderberg Garden ...

Indian basketball league Indian National Basketball League (INBL)SportBasketballFounded2021; 3 years ago (2021)CEOParveen Batish Rupinder BrarMottoDONE. HIDING.No. of teams6CountryIndiaMost recentchampion(s)Chennai Heat (1st title) (2022)TV partner(s)YouTube FanCodeOfficial websitehttps://inbl.basketball/ The Indian National Basketball League (INBL) is the open-entry off-season developmental basketball league in India, organized and by the HAI and Basketball Federation of In...

Kementerian Agama Republik IndonesiaLambang Kementerian AgamaBendera Kementerian AgamaGambaran umumDibentuk3 Januari 1946; 78 tahun lalu (1946-01-03)Dasar hukum pendirianPeraturan Presiden Nomor 12 Tahun 2023SloganIkhlas BeramalPegawai235.343 per 2020Alokasi APBNRp67,1 Triliun Susunan organisasiMenteriYaqut Cholil QoumasWakil MenteriSaiful Rahmat DasukiSekretaris JenderalNizar AliInspektur JenderalFaisal Ali Hasyim Direktur JenderalPendidikan IslamMuhammad Ali RamdhaniPenyelenggaraan Haj...

Canadian ice hockey player, executive (b. 1969) For the Australian author and journalist, see Brendan Shanahan (author). Ice hockey player Brendan Shanahan Hockey Hall of Fame, 2013 Shanahan in 2020Born (1969-01-23) January 23, 1969 (age 55)Mimico, Ontario, CanadaHeight 6 ft 3 in (191 cm)Weight 220 lb (100 kg; 15 st 10 lb)Position Left wingShot RightPlayed for New Jersey DevilsSt. Louis BluesDüsseldorfer EGHartford WhalersDetroit Red WingsNew York Rang...

Aeroméxico IATA ICAO Kode panggil AM AMX AEROMEXICO Didirikan14 September 1934 (sebagai Aeronaves de México S.A. de C.V.)Mulai beroperasi14 September 19341 Oktober 1988 (sebagai Aerovias de México S.A. de C.V.)PenghubungBandara Kota MeksikoKota fokusBandara GuadalajaraBandara MonterreyProgram penumpang setiaClub PremierLounge bandaraSalón PremierAliansiSkyTeamArmada88Tujuan89SloganMexico's Global AirlinePerusahaan indukGrupo AeroméxicoKantor pusatKota Meksiko, MeksikoTokoh utamaAndrés C...
LEGO Star Wars: Il risveglio della ForzavideogiocoTitolo originaleLego Star Wars: The Force Awakens PiattaformaMicrosoft Windows, Nintendo 3DS, macOS, PlayStation 3, PlayStation 4, PlayStation Vita, Wii U, Xbox 360, Xbox One, iOS, Android Data di pubblicazione 28 giugno 2016 28 giugno 2016 28 giugno 2016OS X: 30 giugno 2016Android: 27 luglio 2016 GenereAzione, avventura OrigineRegno Unito SviluppoTraveller's Tales PubblicazioneWarner Bros. Interacti...

Policy opposing discrimination against non-Malay populations This article is part of a series on thePolitics ofMalaysia Head of State Yang di-Pertuan Agong Ibrahim Iskandar Conference of Rulers Legislature Parliament of Malaysia 15th Parliament Senate (Dewan Negara) President Wan Junaidi Tuanku Jaafar House of Representatives (Dewan Rakyat) Speaker Johari Abdul Leader of the Government Anwar Ibrahim Leader of the Opposition Hamzah Zainudin Executive Cabinet Prime Minister Anwar Ibrahim Civil ...

Que mon cœur lâche Logo de la pochette du single. Single de Mylène Farmerextrait de l'album Dance Remixes Sortie 16 novembre 1992 Durée 4:05 Genre Pop, Variété française Format 45 tours, Maxi 45 tours, CD Single, Cassette Auteur Mylène Farmer Compositeur Laurent Boutonnat Label Polydor, Toutankhamon Singles de Mylène Farmer Beyond My Control(1992) XXL(1995)modifier Que mon cœur lâche est une chanson de Mylène Farmer, sortie en single le 16 novembre 1992. Ce titre, dont ...

Artikel ini bukan mengenai Gunung Bromo. Bahasa Oromo Afaan Oromoo Dituturkan diEthiopia, KenyaWilayahOromiaEtnisOromoPenutur34 juta penduduk di Ethiopia (2015)[1]500 di Kenya (sensus 2015), 42 di Somalia (sensus 2015)[1] Rincian data penutur Jumlah penutur beserta (jika ada) metode pengambilan, jenis, tanggal, dan tempat.[2] 24.000.000 (2007, Bahasa ibu)36.000.000 ±1000000 Rumpun bahasaAfroasiatik KushitikDataran Rendah TimurOromoidOromo Sistem penulisanLat...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (مارس 2016) This list divides the world using the seven-continent model, with islands grouped into adjacent continents. The continents are: إفريقيا آسيا أوروبا أمريكا الشمالية ...
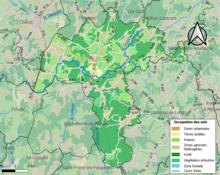
Peta infrastruktur dan tata guna lahan di Komune Bussière-Galant. = Kawasan perkotaan = Lahan subur = Padang rumput = Lahan pertanaman campuran = Hutan = Vegetasi perdu = Lahan basah = Anak sungaiBussière-Galant merupakan sebuah komune di departemen Haute-Vienne di Prancis. Lihat pula Komune di departemen Haute-Vienne Referensi INSEE lbsKomune di departemen Haute-Vienne Aixe-sur-Vienne Ambazac Arnac-la-Poste Augne Aureil Azat-le-Ris Balledent ...

Cet article est une ébauche concernant le Guyana. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Carte des colonies néerlandaises de la Côte Sauvage (Amérique du Sud) autour de 1800. De gauche à droite : Pomeroon, Essequibo, Démérara et Berbice. Berbice (/bɛʁ.bis/) était une ancienne colonie néerlandaise située le long du fleuve Berbice sur la Côte Sauvage sud-américaine, dans l'actuel Guyana...

Conference between nations in the Commonwealth 1926 Imperial ConferenceThe King (front, centre) with his prime ministers. Standing (left to right): W.S. Monroe (Newfoundland), Gordon Coates (New Zealand), Stanley Bruce (Australia), J. B. M. Hertzog (Union of South Africa), W. T. Cosgrave (Irish Free State). Seated: Stanley Baldwin (United Kingdom), King George V, William Mackenzie King (Canada).Host country United KingdomDate19 October 1926–23 November 1926CitiesLondonHeads of State or Gove...

منتخب سويسرا لهوكي الجليد للناشئين البلد سويسرا ألوان الفريق رمز IIHF SUI مشاركة دولية الاتحاد السوفيتي 18 – 1 سويسرا (كورنوال (أونتاريو)، أونتاريو، كندا؛ 22 ديسمبر 1977) أكبر فوز سويسرا 20 – 7 النمسا (سابورو، اليابان؛ 24 مارس 1985) أكبر هزيمة فنلندا 19 – 1...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (يوليو 2016) سباق الأومنيوم للسيدات في بطولة الدراجات على المضمار الاختصاص سباق الدراجات على المضمار المنظَم الاتحاد ...

ДеревняЛедухи 56°54′29″ с. ш. 53°50′30″ в. д.HGЯO Страна Россия Субъект Федерации Удмуртская Республика Муниципальный округ Воткинский История и география Высота центра 115 м Население Население ↗11[1] человек (2012) Цифровые идентификаторы Почтовый индекс 4274...

لمعانٍ أخرى، طالع الحميراء (توضيح). الحميراء (محلة) تقسيم إداري البلد اليمن المحافظة محافظة إب المديرية مديرية السبرة العزلة عزلة عينان القرية قرية غضار السكان التعداد السكاني 2004 السكان 55 • الذكور 26 • الإناث 29 • عدد الأسر 4 • عدد المساكن 4 معلومات أ...

Airport in NhulunbuyGove AirportMain terminal entrance (2002)IATA: GOVICAO: YPGVSummaryAirport typePublicOwnerNhulunbuy CorporationOperatorNhulunbuy CorporationServesGove Peninsula, Northern Territory, AustraliaLocationNhulunbuyElevation AMSL205 ft / 62 mCoordinates12°16′12″S 136°49′06″E / 12.27000°S 136.81833°E / -12.27000; 136.81833MapYPGVLocation in the Northern TerritoryRunways Direction Length Surface m ft 13/31 2,208 7,244 Asphalt Stat...

British Rail discount card Network Card redirects here. For the computer networking component, see Network interface controller. Network RailcardProduct typePublic transportCountryUnited KingdomIntroduced1986Related brandsNational Rail EnquiriesBritish RailMarketsUnited KingdomWebsitewww.network-railcard.co.uk The earliest version of the Network Card, issued manually rather than through an APTIS machine. The first APTIS version of the Network Card. This was used from the start of the APTIS er...




























