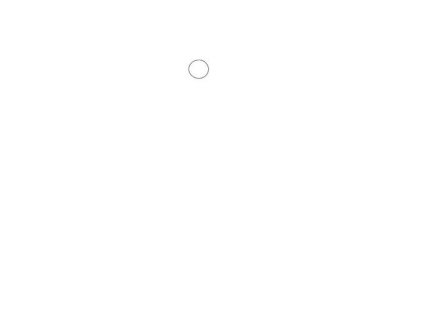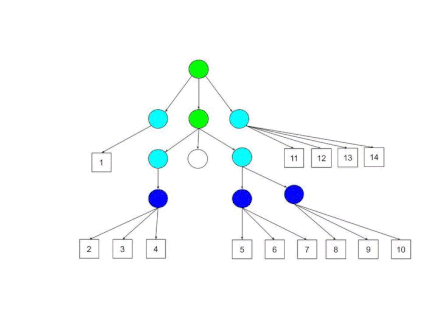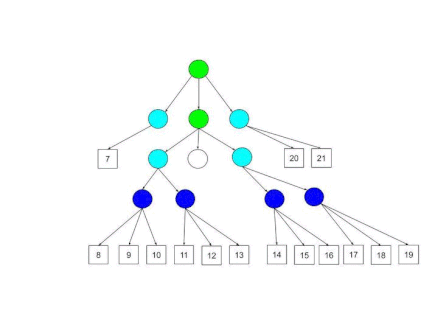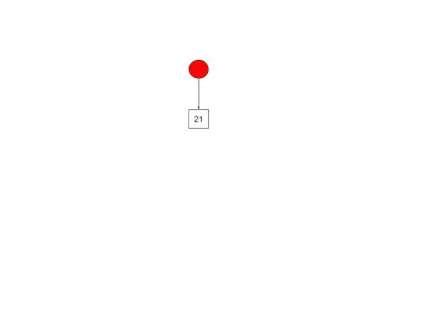
Finger tree
|
Read other articles:

All SaintsInformasi latar belakangNama lainAll Saints 1.9.7.5 (1993–95)AsalLondon, InggrisGenre Pop R&B elektronika Tahun aktif 1993–2001 2006–2008 2013–sekarang Label ZTT London Parlophone Situs weballsaintsofficial.co.ukAnggotaMelanie BlattShaznay LewisNicole AppletonNatalie AppletonMantan anggotaSimone Rainford All Saints adalah sebuah grup musik asal Inggris-Kanada yang dibentuk tahun 1993.[1] Mereka didirikan sebagai All Saints 1.9.7.5 oleh anggota Melanie Blatt, Shaz...

Demokrat Kristen adalah sebutan bagi para penganut paham demokrasi Kristen sebagai ideologi politik yang ide utamanya adalah mengkonsepsikan penyatuan antara konservatisme religius, khususnya Kristen Katholik Roma dengan demokrasi, dan liberalisme. Paham ini berkembang di Eropa Barat, khususnya di negara-negara daratan Eropa – tidak termasuk Inggris - seperti Jerman dan Prancis.[1][2][3][4][5] Latar Belakang Pada 1945, pasca Perang Dunia II, ideologi ...

Untuk kegunaan lain, lihat Palu (disambiguasi). Palu Palu, pemukul, tukul, atau martil adalah alat yang digunakan untuk memberikan tumbukan kepada benda. Palu umum digunakan untuk memaku, memperbaiki suatu benda, penempaan logam dan menghancurkan suatu objek. Palu dirancang untuk tujuan tertentu dengan variasi dalam bentuk dan struktur. Bentuk umum palu terdiri dari gagang palu dan kepala palu, dengan sebagian besar berat berada di kepala palu. Desain dasar palu agar mudah digunakan, tetapi a...

Frank RiceKartu lobi menampilkan Rice (ketiga dari kiri) dalam Spook Ranch (1925)Lahir(1892-05-13)13 Mei 1892Muskegon, Michigan, Amerika SerikatMeninggal9 Januari 1936(1936-01-09) (umur 43)Los Angeles, California, Amerika SerikatTahun aktif1912-1936 Frank Rice (13 Mei 1892 – 9 Januari 1936) adalah seorang pemeran film Amerika Serikat. Ia tampil dalam lebih dari 120 film antara 1912 dan 1936. Ia lahir di Muskegon, Michigan, dan meninggal di Los Angeles, California ak...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Maret 2016. SMP Negeri 4 TulakanInformasiDidirikan1998Jumlah kelas13Jurusan atau peminatanumumRentang kelasVII, VIII, IXKurikulumKurikulum Tingkat Satuan PendidikanJumlah siswa447AlamatLokasiDesa Wonosidi, Kecamatan Tulakan, Pacitan, Jawa TimurMoto SMP Neg...

Ini adalah nama Tionghoa; marganya adalah Oei. Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Oei Tjong Hauw – berita · surat kabar · buku · cendekiawan · JSTOR Oei Tjong Hauw adalah ketua partai Chung Hwa Hui (CHH), partai kaum peranakan Tionghoa di...

Racing team This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Wayne Taylor Racing – news · newspapers · books · scholar · JSTOR (November 2020) (Learn how an...

American racecar driver Hurley HaywoodHaywood in 1994Nationality AmericanBorn (1948-05-04) May 4, 1948 (age 75)Chicago, Illinois24 Hours of Le Mans careerYears1977–1983, 1985–1987, 1990–1991, 1993–1994Best finish1st (1977, 1983, 1994)Class wins3 (1977, 1983, 1994) The Porsche 936 which Hurley Haywood drove to victory at the 1977 24 Hour of Le Mans Harris Hurley Haywood (born May 4, 1948)[1] is a retired American race car driver. Haywood has won multiple events, inclu...

Inverse image of zero under a homomorphism For other uses, see Kernel (disambiguation). In mathematics, the kernel of a linear map, also known as the null space or nullspace, is the part (or linear subspace) of the domain which the map maps to the zero vector.[1] That is, given a linear map L : V → W between two vector spaces V and W, the kernel of L is the vector space of all elements v of V such that L(v) = 0, where 0 denotes the zero vector in W,[2] or more symbolica...

Ini adalah sebuah nama Indonesia yang tidak menggunakan nama keluarga. Nama Soekarnoputri adalah sebuah patronimik. Sukmawati Soekarnoputri Informasi pribadiLahirDiah Mutiara Sukmawati Sukarnoputri26 Oktober 1951 (umur 72)Jakarta, IndonesiaPartai politikPNIMSuami/istriMangkunegara IX (m. 1974; c. 1984)Anak3, termasuk GPH Paundrakarna Sukmaputra JiwanegaraOrang tuaSoekarnoFatmawatiPekerjaanSenimanpolitikuspengusahaSunting kotak info ...

American football player (born 1984) For similarly-named American football players, see Joe Thomas (wide receiver) and Joe Thomas (linebacker). American football player Joe ThomasThomas with the Browns in 2015Munich RavensPosition:Offensive line coachPersonal informationBorn: (1984-12-04) December 4, 1984 (age 39)Brookfield, Wisconsin, U.S.Height:6 ft 6 in (1.98 m)Weight:312 lb (142 kg)Career informationHigh school:Brookfield Central (Brookfield, Wisconsin)Colleg...

この記事は検証可能な参考文献や出典が全く示されていないか、不十分です。出典を追加して記事の信頼性向上にご協力ください。(このテンプレートの使い方)出典検索?: コルク – ニュース · 書籍 · スカラー · CiNii · J-STAGE · NDL · dlib.jp · ジャパンサーチ · TWL(2017年4月) コルクを打ち抜いて作った瓶の栓 コルク(木栓、�...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Oktober 2022. Geologi fisik adalah salah satu cabang ilmu geologi yang mempelajari Bumi dari sifat fisiknya. Ruang lingkup geologi fisik meliputi bahan pembentuk Bumi, atmosfer Bumi, hidrosfer serta proses-proses yang menerima pengaruh dari energi surya dan gravitas...

土库曼斯坦总统土库曼斯坦国徽土库曼斯坦总统旗現任谢尔达尔·别尔德穆哈梅多夫自2022年3月19日官邸阿什哈巴德总统府(Oguzkhan Presidential Palace)機關所在地阿什哈巴德任命者直接选举任期7年,可连选连任首任萨帕尔穆拉特·尼亚佐夫设立1991年10月27日 土库曼斯坦土库曼斯坦政府与政治 国家政府 土库曼斯坦宪法 国旗 国徽 国歌 立法機關(英语:National Council of Turkmenistan) ...

土库曼斯坦总统土库曼斯坦国徽土库曼斯坦总统旗現任谢尔达尔·别尔德穆哈梅多夫自2022年3月19日官邸阿什哈巴德总统府(Oguzkhan Presidential Palace)機關所在地阿什哈巴德任命者直接选举任期7年,可连选连任首任萨帕尔穆拉特·尼亚佐夫设立1991年10月27日 土库曼斯坦土库曼斯坦政府与政治 国家政府 土库曼斯坦宪法 国旗 国徽 国歌 立法機關(英语:National Council of Turkmenistan) ...

Spanish television series (2001–2023) This article is about the Spanish TV series. For Argentinian series, see Cuéntame cómo pasó (Argentina). Cuéntame cómo pasóAlso known as Cuéntame Remember When Genre Historical drama Comedy drama Created byMiguel Ángel Bernardeau [es]Starring Imanol Arias Ana Duato Ricardo Gómez María Galiana Pablo Rivero Irene Visedo Narrated byCarlos HipólitoOpening themeCuéntame [es]Country of originSpainOriginal languageSpanishN...

本表是動態列表,或許永遠不會完結。歡迎您參考可靠來源來查漏補缺。 潛伏於中華民國國軍中的中共間諜列表收錄根據公開資料來源,曾潛伏於中華民國國軍、被中國共產黨聲稱或承認,或者遭中華民國政府調查審判,為中華人民共和國和中國人民解放軍進行間諜行為的人物。以下列表以現今可查知時間為準,正確的間諜活動或洩漏機密時間可能早於或晚於以下所歸�...

Singkatan stasiun ini bukan berarti Rupiah. Artikel ini bukan mengenai Stasiun Indramayu. Stasiun Indralaya K02 Peron Stasiun Indralaya dengan KA KertalayaLokasiJalan Raya Palembang-PrabumulihTanjung Pering, Indralaya, Ogan Ilir, Sumatera Selatan 30813IndonesiaKoordinat3°12′43″S 104°38′17″E / 3.21194°S 104.63806°E / -3.21194; 104.63806Koordinat: 3°12′43″S 104°38′17″E / 3.21194°S 104.63806°E / -3.21194; 104.63806Ketinggian...

موزوراس الإحداثيات 35°32′00″N 24°09′00″E / 35.53333333°N 24.15°E / 35.53333333; 24.15 تقسيم إداري البلد اليونان[1] عدد السكان عدد السكان 186 (2021)949 (2001)466 (1991)134 (2011) رمز جيونيمز 256660 تعديل مصدري - تعديل موزوراس (باليونانية: Μουζουράς) هي مدينة في خانية في اليون�...

هيركوليس الإحداثيات 38°01′02″N 122°17′19″W / 38.017222222222°N 122.28861111111°W / 38.017222222222; -122.28861111111 [1] تاريخ التأسيس 1900 تقسيم إداري البلد الولايات المتحدة[2][3] التقسيم الأعلى مقاطعة كونترا كوستا خصائص جغرافية المساحة 51.757738 كيلومتر مربع47.08...