Credal set
|
Read other articles:

Pertanian Umum Agribisnis Agroindustri Agronomi Ilmu pertanian Jelajah bebas Kebijakan pertanian Lahan usaha tani Mekanisasi pertanian Menteri Pertanian Perguruan tinggi pertanian Perguruan tinggi pertanian di Indonesia Permakultur Pertanian bebas ternak Pertanian berkelanjutan Pertanian ekstensif Pertanian intensif Pertanian organik Pertanian urban Peternakan Peternakan pabrik Wanatani Sejarah Sejarah pertanian Sejarah pertanian organik Revolusi pertanian Arab Revolusi pertanian Inggris Revo...

City of Michoacán, Mexico This article is about the Mexican City. For other uses, see Morelia (disambiguation). City in Michoacán, MexicoMoreliaCityFrom top to bottom, from left to right: View of the city, the Cathedral of Morelia, the Aqueduct of Morelia, the Government Palace of Michoacán, the main square, the Federal Palace, the Monument to José María Morelos y Pavón and the Clavijero Cultural Center FlagCoat of armsMoreliaShow map of MichoacánMoreliaShow map of MexicoCoordinates: 1...

AbunKecamatanAbunPeta lokasi Kecamatan AbunTampilkan peta Semenanjung Kepala BurungAbunAbun (Indonesia)Tampilkan peta IndonesiaKoordinat: 0°23′S 132°40′E / 0.383°S 132.667°E / -0.383; 132.667Koordinat: 0°23′S 132°40′E / 0.383°S 132.667°E / -0.383; 132.667Negara IndonesiaProvinsiPapua Barat DayaKabupatenTambrauwLuas[1] • Total845,91 km2 (326,61 sq mi)Populasi (Desember 2022)[2]&...

Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article ne cite pas suffisamment ses sources (mars 2024). Si vous disposez d'ouvrages ou d'articles de référence ou si vous connaissez des sites web de qualité traitant du thème abordé ici, merci de compléter l'article en donnant les références utiles à sa vérifiabilité et en les liant à la section « Notes et références ». En pratique : Quelles sources sont attendues ? Comm...

Tombes peintes de LiaoyangPrésentationType Site archéologiquePatrimonialité Site national majeur (1961)LocalisationLocalisation Liaoyang, Liaoning ChineCoordonnées 41° 18′ 58″ N, 123° 10′ 14″ Emodifier - modifier le code - modifier Wikidata Les tombes peintes de Liaoyang sont des grandes tombes en pierre possédant plusieurs chambres funéraires célèbres pour leurs fresques et datant de la fin de la dynastie Han (25-220) et du royaume de...

Esperance Sportive De TunisNama lengkapEsperance Sportive de TunisJulukanMkachkha, Blood & Gold, TarajiBerdiri15 Januari 1919StadionStade Olympique de RadesTunis, Tunisia(Kapasitas: 60,000)Ketua Hamdi MeddebManajer Maher Kanzari Skander Kasri (asisten pelatih)LigaCLP-12020/21Juara Kostum kandang Kostum ketiga Espérance's active sections Sepak bola Bola Tangan Bola Voli Rugby Renang Gulat Tinju Judo Espérance Sportive de Tunis (Arab: الترجي الرياضي التونسيcode: ar is ...

Katarzyna Sobieska Katarzyna Sobieska (Zoločiv, 7 gennaio 1634 – Varsavia, 29 settembre 1694) è stata una nobile polacca, sorella del re Giovanni III di Polonia. Indice 1 Biografia 2 Matrimoni 2.1 Primo Matrimonio 2.2 Secondo Matrimonio 3 Morte 4 Note 5 Altri progetti 6 Collegamenti esterni Biografia Katarzyna era la figlia di Jakub Sobieski, Voivoda di Rutenia nonché castellano di Cracovia, e di sua moglie, Zofia Teofila Daniłowiczówna, figlia di Jan Daniłowicz. Subito dopo la sua na...

In this Dutch name, the surname is van Bleiswijk, not Bleiswijk. Portrait of Pieter van Bleiswijk (between 1750 and 1789) Pieter van Bleiswijk (1724, Delft – 29 October 1790, The Hague[1]) was grand pensionary of Holland from 1 December 1772 to November 1787. He was an opponent of Duke Louis Ernest of Brunswick-Lüneburg, the main adviser of Prince William V of Orange. He was deposed during the Prussian invasion of the United Provinces in 1787. References ^ Mr. drs. Dirk van Dui...

Election for the Governor of Nevada 1874 Nevada gubernatorial election ← 1870 3 November 1874 1878 → Nominee Lewis R. Bradley John Clark Hazlett Party Democratic Republican Popular vote 10,310 7,785 Percentage 56.98% 43.02% Governor before election Lewis R. Bradley Democratic Elected Governor Lewis R. Bradley Democratic Elections in Nevada Federal government U.S. President 1864 1868 1872 1876 1880 1884 1888 1892 1896 1900 1904 1908 1912 Dem GOP 1916 Dem GOP 1...

Football club in Spain active between 1899 and 1928 Football clubCatalà Futbol ClubShort nameCatalà FCFounded17 December 1899; 124 years ago (1899-12-17)Dissolvedlate 1920sGroundVelódromo de la BonanovaLeagueCatalan championship Home colours Away colours Català Futbol Club, and later Català Sport Club, was a football team based in Barcelona, Spain, which existed from 1899 until its dissolution in the late 1920s. It was one of the most important entities in the amateur b...
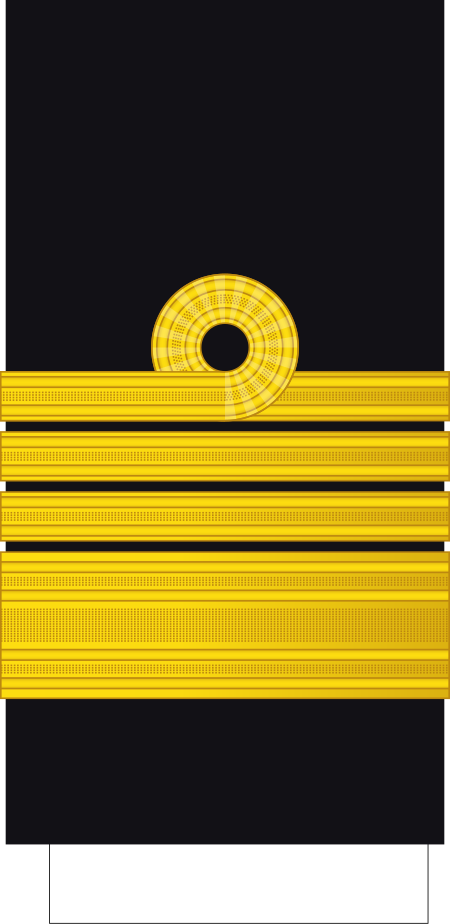
Overview of the ranks and insignia of the Pakistan Navy The Pakistan Navy ranks and insignia are the military insignia used by the Pakistan Navy. They are used to identify their roles as commanding officers and varies with their responsibilities.[1] Pakistan shares a rank structure similar to that of the United Kingdom.[2] Officers Rank group General/flag officers Senior officers Junior officers Officer cadet Pakistan Navy[1]vte Admiralایڈمرل Vice admiralو...

Cet article est une ébauche concernant une localité italienne et le Latium. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Antrodoco Piazza del popolo Administration Pays Italie Région Latium Province Rieti Code postal 02013 Code ISTAT 057003 Code cadastral A315 Préfixe tel. 0746 Démographie Gentilé antrodocani Population 2 442 hab. (30-04-2019[1]) Densité 39 hab./km2 Géograph...

第三十二届夏季奥林匹克运动会柔道比賽比賽場館日本武道館日期2021年7月24日至31日項目數15参赛选手393(含未上场5人)位選手,來自128(含未上场4队)個國家和地區← 20162024 → 2020年夏季奥林匹克运动会柔道比赛个人男子女子60公斤级48公斤级66公斤级52公斤级73公斤级57公斤级81公斤级63公斤级90公斤级70公斤级100公斤级78公斤级100公斤以上级78公斤以上级团体混...

Wadi Hammamat, (bahasa Arab: وادي الحمامات, transliterasi huruf Latin menjadi Wādi al Hamāmāt; bahasa Koptik: ⲣⲱϩⲉⲛⲧⲟⲩ, transliterasi huruf Latin menjadi rōhentouw), adalah sebuah wadi atau sungai ephemeral di sebelah timur negara Mesir. Wadi ini terbentang di sepanjang Gurun Timur dari Kota Qift atau Koptos di Kegubernuran Qina sampai Kota Al Qusair di Kegubernuran Laut Merah. Meskipun kota Qift dan Qina termasuk bagian dari Cekungan Sungai Nil, sungai ini ...

ٱلْحَسَنُ بْنُ عَلِيِّ بْنِ أَبِي طَالِبٍ تخطيط لاسم الحَسَن ملحق بالترضي عنه سِبْطُ رَسُولِ ٱللّٰهِ وَحَفِيدُهُ وَرَيْحَانَتُهُ، سَيِّدُ شَبَابِ أَهْلِ ٱلْجَنَّةِ الكنية أبو محمّد الولادة 15 رمضان 3 هـ، الموافق 4 مارس 625مالمدينة المنورة الوفاة 49 هـ، أو 50 هـ، أو 51 هـالمد�...

Vous lisez un « bon article » labellisé en 2012. Warcraft IITides of DarknessDéveloppeur Blizzard Entertainment, Climax GroupÉditeur Blizzard Entertainment, Ubisoft, Electronic ArtsDate de sortie Décembre 1995Microsoft Windows, Mac OS AN : Décembre 1995EUR : 1996 Saturn, PlayStation AN/EUR : 1997 Genre Stratégie en temps réelMode de jeu Un joueur, multijoueurPlate-forme Ordinateur(s) :AmigaOS 4, DOS, Linux, Mac OS, WindowsConsole(s) :Saturn, PlaySt...

Color trend forecasting and consulting organization Color Association of the United StatesFormerlyTextile Color Card Association of the United States (1915-1955)Company typeIncentive Corporation LimitedIndustryEntertainment GroupPredecessorTextile Color Card Association of the United StatesFounded1914 (as Textile Color Card Association of the United States)1955 (as Color Association of the United States)HeadquartersUnited StatesArea servedWorldwideWebsitewww.colorassociation.com The Color Ass...

Музей органической культуры / Museum of Organic Culture Дата основания 2011 Адрес 140400 МО г. Коломна, Кремль, ул. Казакова дом 10 Коломна, Россия Директор Татьяна Киля Сайт museumart.ru Медиафайлы на Викискладе Объект культурного наследия России регионального значениярег. № 501410192490005 (ЕГРОК...

Эта статья или раздел нуждается в переработке.Пожалуйста, улучшите статью в соответствии с правилами написания статей. Замеча́тельные преде́лы — термины, использующиеся в советских и российских учебниках по математическому анализу для обозначения двух широко изве�...

Charles Dickens Dickens Unterschrift Charles Dickens, 1843 Charles John Huffam Dickens, (als Pseudonym auch Boz; * 7. Februar 1812 in Landport bei Portsmouth, England; † 9. Juni 1870 auf seinem Landsitz Gads Hill Place in Higham bei Rochester, England) war ein englischer Schriftsteller. Ihm wird große literaturgeschichtliche Bedeutung beigemessen. 2015 wählten 82 internationale Literaturkritiker und -wissenschaftler vier seiner Romane zu den bedeutendsten britischen Romanen: David Co...

![{\displaystyle \mathrm {ext} [K(X)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/014d75a3368b5986a2a4932bfe0b606b066d2b34)


![{\displaystyle [{\underline {E}}[f],{\overline {E}}[f]]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/71ef2b73f25f6536deceaef4674c69f512217d0e)
![{\displaystyle {\underline {E}}[f]=\min _{\mu \in K(X)}\int f\,d\mu =\min _{\mu \in \mathrm {ext} [K(X)]}\int f\,d\mu }](https://wikimedia.org/api/rest_v1/media/math/render/svg/501557f82a17cb141018f773d881ea2440f0af43)

![{\displaystyle {\overline {E}}[f]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e2adcb1e5d3a593652c0a5af7f717bb55dea2770)


![{\displaystyle {\underline {E}}[f]=\min _{p\in \mathrm {ext} [K(X)]}\sum _{x}f(x)p(x)}](https://wikimedia.org/api/rest_v1/media/math/render/svg/09a0b54ff4d81feef07b66bf5418696b29b2a5dd)

