Conditional entropy
|
Read other articles:
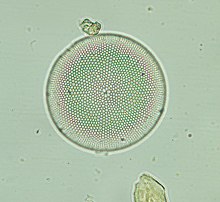
Coscinodiscus Coscinodiscus radiatus Klasifikasi ilmiah (tanpa takson): SAR Superfilum: Heterokonta Filum: Bacillariophyta Kelas: Coscinodiscophyceae Ordo: Coscinodiscales Famili: Coscinodiscaceae Genus: CoscinodiscusEhrenberg, 1839 Spesies lihat teks Coscinodiscus adalah genus diatom dalam keluarga Coscinodiscaceae. Genus ini adalah tipe genus dari keluarganya. Coscinodiscus memiliki bentuk bulat dengan diameter lebih dari 400 mikrometer[1] Referensi ^ Nontji, Anugerah (2008). Plank...

Mobile ESPN est un service américains d'informations sportives sur téléphone mobile. Il remplace l'opérateur de téléphonie mobile virtuel homonyme qui fait lui suite à un service identique, nommé ESPN Mobile, proposé par le réseau de chaînes télévisées et de radio ESPN. Ce service a été révélé en octobre 2004 et officialisé le 1er décembre 2004 sous le nom ESPN Mobile[1] comme simple fil d'information disponible sur les téléphones mobiles puis doit devenir un opérateu...

Dewan Perwakilan Rakyat Daerah Kabupaten Ciamis ᮓᮦᮝᮔ᮪ ᮕᮀᮝᮊᮤᮜ᮪ ᮛᮠᮚᮒ᮪ ᮓᮆᮛᮂ ᮊᮘᮥᮕᮒᮦᮔ᮪ ᮎᮤᮃᮙᮤᮞ᮪Déwan Pangwakil Rahayat Daérah Kabupatén CiamisDewan Perwakilan RakyatKabupaten Ciamis2019-2024JenisJenisUnikameral SejarahSesi baru dimulai5 Agustus 2019PimpinanKetuaNanang Permana, S.H. (PDI-P) sejak 24 September 2019 Wakil Ketua IH. Dede Herli, S.Pt., M.M. (PKS) sejak 24 September 2019 Wakil Ketua IIHeri Rafni Kotari, S....

Cet article est une ébauche concernant le droit français. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Article 74 de la Constitution du 4 octobre 1958 Données clés Présentation Pays France Langue(s) officielle(s) Français Type Article de la Constitution Adoption et entrée en vigueur Législature IIIe législature de la Quatrième République française Gouvernement Charles de Gaulle (3e) Promulgation 4...

List of characters in the Pokémon franchise This article is about the human characters in the Pokémon video game franchise. For the creatures known as Pokémon, see List of Pokémon. For the characters in the anime TV series, see List of Pokémon anime characters. For the characters in the Pokémon Adventures manga series, see List of Pokémon Adventures characters. This article needs additional citations for verification. Please help improve this article by adding citations to reliable sou...

This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately from the article and its talk page, especially if potentially libelous.Find sources: Gabi Tóth – new...

Artikel ini bukan mengenai Trans Semanggi Suroboyo. Layanan ini terkoneksi dengan moda angkutan perkotaan non bus seperti angkutan kota (bemo) dan Wirawiri Suroboyo di beberapa titik lokasi pada kawasan perkotaan Surabaya. Suroboyo BusSebuah unit koridor R5/R6 Suroboyo Bus melintasi Halte Gunung Anyar pada 17 Juni 2021.Didirikan7 April 2018Kantor pusatGedung PNR Mayjen Sungkono,Jalan Mayjen Sungkono Nomor 122, Kelurahan Gunungsari, Kecamatan Dukuh Pakis, Kota Surabaya, Provinsi Jawa Timur, Ko...

Album by Enrico Rava Enrico Rava QuartetStudio album by the Enrico Rava QuartetReleased1978RecordedMarch 1978StudioTonstudio BauerLudwigsburg, West GermanyGenreJazzLength42:08LabelECM 1122ProducerManfred EicherEnrico Rava chronology The Plot(1976) Enrico Rava Quartet(1978) Ah(1979) Enrico Rava Quartet is an album by the Enrico Rava Quartet, recorded in March 1978 and released on ECM later that year. The quartet features trombonist Roswell Rudd and rhythm section Jean-François Jenny-Clark...

910 Tegal Parang Halte TransjakartaHalte arah Pancoran Barat, Februari 2024LetakKotaJakarta SelatanDesa/kelurahanKuningan Timur, Setiabudi (sisi timur)Kuningan Barat, Mampang Prapatan (sisi barat)Kodepos12710AlamatJalan Jenderal Gatot SubrotoKoordinat6°14′21″S 106°49′51″E / 6.2393°S 106.8308°E / -6.2393; 106.8308Koordinat: 6°14′21″S 106°49′51″E / 6.2393°S 106.8308°E / -6.2393; 106.8308Desain HalteStruktur BRT, media...

Fotomikrograf dibuat dengan mikroskop pemindai elektron (SEM) serta detektor pencar belakang: penampang partikel abu terbang pada perbesaran 750xFotomikrograf dibuat dengan mikroskop pemindai elektron (SEM) pada perbesaran 2000x Abu terbang (bahasa Inggris: fly ash) merupakan sisa dari hasil pembakaran batu bara pada pembangkit listrik. Abu terbang mempunyai titik lebur sekitar 1300 °C dan mempunyai kerapatan massa (densitas), antara 2.0 – 2.5 g/cm3. Abu terbang adalah salah satu ...

Town in Utena County, LithuaniaAndrioniškisTown Coat of armsAndrioniškisCoordinates: 55°35′50″N 25°02′40″E / 55.59722°N 25.04444°E / 55.59722; 25.04444Country LithuaniaCounty Utena CountyMunicipalityAnykščiaiPopulation (2011) • Total229Time zoneUTC+2 (EET) • Summer (DST)UTC+3 (EEST) Andrioniškis (Polish: Androniszki) is a town in Anykščiai District Municipality, in Utena County, in northeast Lithuania. According to t...

Highest point in the state of West Virginia Spruce MountainSpruce Mountain (in the distance) beyond the River Knobs (Judy Rocks, foreground) and the Fore Knobs (middle distance)Highest pointPeakSpruce Knob, Pendleton County, West VirginiaElevation4,863 ft (1,482 m)Coordinates38°41′59″N 79°31′58″W / 38.69972°N 79.53278°W / 38.69972; -79.53278DimensionsLength16 mi (26 km)approximatelyGeographySpruce MountainLocation of Spruce Moun...

2015 British television series This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Capital British TV series – news · newspapers · books · scholar · JSTOR (November 2015) (Learn how and when to remove this message) CapitalBased onCapitalby John LanchesterWritten byPeter BowkerDirected byEuros LynStarring To...

1941 film The Bride Wore CrutchesDirected byShepard TraubeScreenplay byEdward VerdierStory byEdward VerdierAlan DradyProduced byLucien HubbardStarringLynne RobertsTed NorthEdgar KennedyRobert ArmstrongLionel StanderRichard LaneCinematographyCharles G. ClarkeEdited byNick DeMaggioMusic byDavid ButtolphProductioncompany20th Century FoxDistributed by20th Century FoxRelease date May 25, 1941 (1941-05-25) Running time55 minutesCountryUnited StatesLanguageEnglish The Bride Wore Crutc...

提示:此条目页的主题不是中國—巴林關係。 關於中華民國與「巴」字國家的外交關係,詳見中巴關係 (消歧義)。 中華民國—巴林關係 巴林 中華民國 代表機構無駐巴林臺北貿易辦事處代表無代表 陳龍錦 大使[註 1][4] 中華民國—巴林關係(阿拉伯语:العلاقات البحرين جمهورية الصين),是指中華民國與巴林王國之間的關係。�...

Cet article est une ébauche concernant une entreprise et l’énergie. Vous pouvez partager vos connaissances en l’améliorant (comment ?). Une page sur une entreprise étant sujette à controverse, n’oubliez pas d’indiquer dans l’article les critères qui le rendent admissible. Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article ne cite pas suffisamment ses sources (décembre 2013). Si vous disposez d'ouvrages ou d'articles de référen...

Bruno ConcaBruno Conca impegnato con la maglia della Triestina nella stagione 1990-1991.Nazionalità Italia Altezza181 cm Peso72 kg Calcio RuoloAllenatore (ex centrocampista) Termine carriera2000 - giocatore CarrieraGiovanili Catanzaro Squadre di club1 1982-1983 Catanzaro1 (0)1983-1988 Carbonia105 (9)[1]1988-1989 Pro Vercelli33 (3)1989-1990 Pavia26 (1)1990-1994 Triestina111 (3)1994-1995 Salernitana10 (0)1995-1996 Atletico Catania35 (0)1996...

1995 2011 Élections sénatoriales de 2004 à Saint-Pierre-et-Miquelon 26 septembre 2004 Type d’élection Élections sénatoriales Postes à élire 1 siège de sénateur Denis Detcheverry – AD Voix au 1er tour 6 15,79 % Voix au 2e tour 19 51,35 % Karine Claireaux – DIA Voix au 1er tour 13 34,21 % Voix au 2e tour 18 48,65 % Marc Plantagenest – DIA Voix au 1er tour 11 28,95 % Yannick Cambray – C...

37°55′48″N 42°16′13″E / 37.93000°N 42.27028°E / 37.93000; 42.27028 محافظة سعرد الإحداثيات 37°55′48″N 42°16′13″E / 37.93°N 42.270277777778°E / 37.93; 42.270277777778 [1] تقسيم إداري البلد تركيا[2][3] التقسيم الأعلى تركيا العاصمة سعرد التقسيمات الإدارية كورتالانس...

1993 video game This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Fatty Bear's Birthday Surprise – news · newspapers · books · scholar · JSTOR (March 2015) (Learn how and when to remove this message) 1993 video gameFatty Bear's Birthday SurpriseCover artDeveloper(s)Humongous EntertainmentPublisher(s)Humongous ...
















![{\displaystyle H(Y|X)=\mathbb {E} [f(X,Y)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6e7cfdc7f8953ec5eed3ea5897b0b24223941d6b)











![{\displaystyle \mathrm {H} (Y):=\mathbb {E} [\operatorname {I} (Y)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f114631caeb95e508a74994486e35e972220b378)





![{\displaystyle E_{X}[\mathrm {H} (y_{1},\dots ,y_{n}\mid X=x)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c42f84b74f174cb4c172b6f91074f65dbd915e40)






![{\displaystyle {\begin{aligned}\mathrm {H} (Y|X)&=\sum _{x\in {\mathcal {X}},y\in {\mathcal {Y}}}p(x,y)\log \left({\frac {p(x)}{p(x,y)}}\right)\\[4pt]&=\sum _{x\in {\mathcal {X}},y\in {\mathcal {Y}}}p(x,y)(\log(p(x))-\log(p(x,y)))\\[4pt]&=-\sum _{x\in {\mathcal {X}},y\in {\mathcal {Y}}}p(x,y)\log(p(x,y))+\sum _{x\in {\mathcal {X}},y\in {\mathcal {Y}}}{p(x,y)\log(p(x))}\\[4pt]&=\mathrm {H} (X,Y)+\sum _{x\in {\mathcal {X}}}p(x)\log(p(x))\\[4pt]&=\mathrm {H} (X,Y)-\mathrm {H} (X).\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/501bd3a915d2218c4464e1ea8cfefc3fba872320)


















![{\displaystyle \mathbb {E} \left[{\bigl (}X-{\widehat {X}}{(Y)}{\bigr )}^{2}\right]\geq {\frac {1}{2\pi e}}e^{2h(X|Y)}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ab916a1ac9b14193bf90b79742772b686bb771c3)