Bicyclic semigroup
|
Read other articles:

The HonourableCarmel SepuloniPotret Sepuloni Wakil Perdana Menteri Selandia Baru ke-20PetahanaMulai menjabat 25 Januari 2023Penguasa monarkiCharles IIIPerdana MenteriChris Hipkins PendahuluGrant RobertsonPenggantiPetahanaMenteri Seni, Budaya, Warisan ke-13Masa jabatan26 Oktober 2017 – 25 Januari 2023Perdana MenteriJacinda Ardern PendahuluJacinda ArdernPenggantiPetahanaAnggota Parlemen Selandia Barudapil KelstonPetahanaMulai menjabat 21 September 2014 PendahuluPosisi dibentu...

László SzabóSzabo pada tahun 1966Nama lengkapLászló SzabóAsal negaraHungariaGelarGrandmasterRating tertinggi2565 (Januari 1973)László Szabó (pengucapan bahasa Hungaria: [ˈsɒboː ˈlaːsloː] 19 Maret 1917 – 8 Agustus 1998) adalah seorang grandmaster catur dari Hungaria. Lahir di Budapest, ia terjun ke kancah percaturan internasional pada tahun 1935, di usia 18, ia memenangkan Kejuaraan Hungaria pertama, sebuah turnamen internasional di Tatatóváros, dan terpi...

« Lermontov » redirige ici. Pour les autres significations, voir Lermontov (homonymie). Mikhaïl Lermontov Mikhaïl Lermontov en 1837. Données clés Nom de naissance Mikhaïl Iourievitch Lermontov Naissance 15 octobre 1814 à Moscou (Empire russe) Décès 27 juillet 1841 (à 26 ans) Mont Machouk, près de Piatigorsk (Empire russe) Activité principale Poète, romancier, dramaturge Auteur Langue d’écriture Russe Mouvement Romantisme, Réalisme Genres Poésie, roman, thé�...

John Sparkman Ketua Komite Senat untuk Hubungan Luar NegeriMasa jabatan3 Januari 1975 – 3 Januari 1979 PendahuluJ. William FulbrightPenggantiFrank ChurchKetua Komite Senat PerbankanMasa jabatan3 Januari 1967 – 3 Januari 1975 PendahuluA. Willis RobertsonPenggantiWilliam ProxmireKetua Komite Senat Usaha KecilMasa jabatan3 Januari 1955 – 3 Januari 1967 PendahuluEdward ThyePenggantiGeorge SmathersMasa jabatan20 Februari 1950 – 3 Januari 1953 PendahuluEd...

Untuk pandangan umum mengenai kitab ini, lihat Injil. Bagian dari seri tentang Pandangan Kristen Kristus Kristologi Nama dan Gelar Riwayat Hidup Injil Keselarasan Injil Petilasan Beribunda Perawan Kelahiran Pembaptisan Karya Pelayanan Khotbah di Bukit Mukjizat Perumpamaan Penistaan Penyaliban Penguburan Kebangkitan Kenaikan Ketaatan Bersemayam di Surga Perantaraan Kedatangan Ke-2 Relikui Isa (Pandangan Islam) Almasih Injil Maryam Hawariyun Wafat Almahdi Hari Kiamat Pusara Latar Belakang Latar...

Batalyon Infanteri Raider Khusus 753/Arga Vira TamaLambang Yonif 753/Arga Vira TamaDibentuk17 Februari 1970NegaraIndonesiaCabangInfanteri RaiderTipe unitSatuan TempurBagian dariKorem 173/Praja Vira BrajaMarkasNabire, Papua TengahJulukanKsatria Walet HitamMotoArga Vira TamaBaretHijau LumutMaskotBurung Walet HitamUlang tahun17 Februari Batalyon Infanteri Raider Khusus 753/Arga Vira Tama atau Yonif RK 753/AVT adalah sebuah batalyon infanteri Tentara Nasional Indonesia (TNI) yang berada di bawah ...

В Википедии есть статьи о других людях с такой фамилией, см. Колчинский. Эдуард Израилевич Колчинский Дата рождения 16 сентября 1944(1944-09-16)[1] Место рождения Карталы, Челябинская область, РСФСР, СССР Дата смерти 24 января 2020(2020-01-24) (75 лет) Страна СССР Россия Научная сф�...

Questa voce sull'argomento stagioni delle società calcistiche italiane è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Voce principale: Delfino Pescara 1936. Società Sportiva PescaraStagione 1942-1943Sport calcio Squadra Pescara Allenatore Luigi Ferrero Presidente Vittorio Angelo Vetta Serie B9º posto. Maggiori presenzeCampionato: Miglio (33) Miglior marcatoreCampionato: Guarnieri (11) 1941-194...

此条目序言章节没有充分总结全文内容要点。 (2019年3月21日)请考虑扩充序言,清晰概述条目所有重點。请在条目的讨论页讨论此问题。 哈萨克斯坦總統哈薩克總統旗現任Қасым-Жомарт Кемелұлы Тоқаев卡瑟姆若马尔特·托卡耶夫自2019年3月20日在任任期7年首任努尔苏丹·纳扎尔巴耶夫设立1990年4月24日(哈薩克蘇維埃社會主義共和國總統) 哈萨克斯坦 哈萨克斯坦政府...

Armed conflict between the United States and Mexico from 1846 to 1848 Mexican–American WarClockwise from top: Winfield Scott entering Plaza de la Constitución after the Fall of Mexico City, U.S. soldiers engaging the retreating Mexican force during the Battle of Resaca de la Palma, U.S. victory at Churubusco outside of Mexico City, Marines storming Chapultepec castle under a large U.S. flag, Battle of Cerro GordoDateApril 25, 1846 – February 2, 1848 (1846-04...

Award ceremony for films of 1984 57th Academy AwardsDateMarch 25, 1985SiteDorothy Chandler PavilionLos Angeles, California, U.S.Hosted byJack LemmonProduced byGregory PeckRobert WiseLarry GelbartGene AllenDirected byMarty PasettaHighlightsBest PictureAmadeusMost awardsAmadeus (8)Most nominationsAmadeus and A Passage to India (11)TV in the United StatesNetworkABCDuration3 hours, 10 minutes ← 56th Academy Awards 58th → The 57th Academy Awards were presented March 25, 1985,...

معركة حصن بورتوفيق إستسلام القائد الإسرائيلي لنقطة بورتوفيق الحصينة بعد الموقعة 1973 معلومات عامة التاريخ 6 أكتوبر، 1973 الموقع سيناء، مصر النتيجة انتصار مصري وأستيلائها علي النقطة - أستسلام القوة الإسرائيلية المتحاربون مصر إسرائيل القادة صلاح عبد الحليمعلي المزاحي �...

Lenovo Group LimitedLogo sejak tahun 2015Kantor pusat Lenovo di Distrik Haidian, BeijingNama asli联想集团有限公司Nama latinLiánxiǎng Jítuán Yǒuxiàn GōngsīJenisPublikKode emitenSEHK: 992Merah Muda OTC: LNVGYIndustriPerangkat keras komputerElektronikDidirikan1 November 1984; 39 tahun lalu (1984-11-01) (sebagai Legend 联想)BeijingPendiriLiu ChuanzhiKantorpusatBeijing, Tiongkok(kantor pusat global)[1][2] Hong Kong, Tiongkok(kantor pusat terdaftar) Morrisvill...

Cari artikel bahasa Cari berdasarkan kode ISO 639 (Uji coba) Cari berdasarkan nilai Glottolog Kolom pencarian ini hanya didukung oleh beberapa antarmuka Halaman rumpun acak Rumpun bahasaBahasa Gallo-Italik Bahasa Gallo-ItaliaPersebaranItalia, San Marino, Swiss, Monako, PrancisPenggolongan bahasaIndo-EropaItalikRomanItalo-BaratGallo-RomanBahasa Gallo-Italik Bahasa Piemonte Bahasa Liguria Bahasa Lombard Bahasa Emilian-Romagnol (Bahasa Venesia)[1][2] Bahasa Ga...

Briscoe County, TexasLokasi di negara bagian TexasLokasi negara bagian Texas di Amerika SerikatDidirikan1876SeatSilvertonWilayah • Keseluruhan902 sq mi (2.336 km2) • Daratan901 sq mi (2.334 km2) • Perairan1 sq mi (3 km2), 0.15%Populasi • (2010)1,637 • Kepadatan3/sq mi (1/km²) Briscoe County adalah county yang terletak di negara bagian Texas, Amerika Serikat. Jumlah penduduk pada tahu...

Railway station in Rzepin, Poland RzepinRailway StationRzepin railway stationGeneral informationLocationRzepin, Lubusz VoivodeshipPolandOperated byPKP Polskie Linie KolejoweLine(s)Warsaw–Kunowice railway Wrocław–Szczecin railway Wierzbno–Rzepin railwayPlatforms6Other informationFare zoneVBB: 5878 (to/from Germany only)[1]HistoryOpened26 June 1870; 154 years ago (1870-06-26)ElectrifiedyesServices Preceding station PKP Intercity Following station Frankfurt (Oder...

M.N. Pokrovsky (1868-1932), sejarawan Soviet. Mikhail Nikolayevich Pokrovsky (bahasa Rusia: Михаи́л Никола́евич Покро́вский, 29 Agustus [K.J.: 17 Agustus] 1868 – 10 April 1932) adalah seorang sejarawan Marxis, revolusioner Bolshevik serta tokoh masyarakat dan politik asal Rusia. Sebagai salah satu sejarawan terlatih secara profesional terawal yang bergabung dengan gerakan revolusioner Rusia, Pokrovsky dipandang sebagai sejarawan Soviet paling berpengaruh pad...

Type of Sari An intricate bandha (Ikat) weave of Sambalpuri sari An intricate weave of Sambalpuri sari pallu A Sambalpuri sari is a traditional handwoven bandha (ikat) sari (locally called sambalpuri bandha sadhi or saree) wherein the warp and the weft are tie-dyed before weaving. It is produced in the Sambalpur, Balangir, Bargarh, Boudh and Sonepur districts of Odisha, India. The sari is a traditional female garment in the Indian subcontinent consisting of a strip of unstitched cloth ranging...

Further information: List of plants of the Sierra Nevada (U.S.) § Alpine Zone, and Ecology of the Sierra Nevada § Alpine Zone The brightly colored sky pilot (Polemonium eximium), considered to be among the most beautiful of the Sierra Nevada wildflowers, grows in very harsh conditions to elevations of 13,000 feet (4,000 m), which is near the upper limit of plant growth in California. The flora of the U.S. Sierra Nevada alpine zone is characterized by small, low growing, cush...
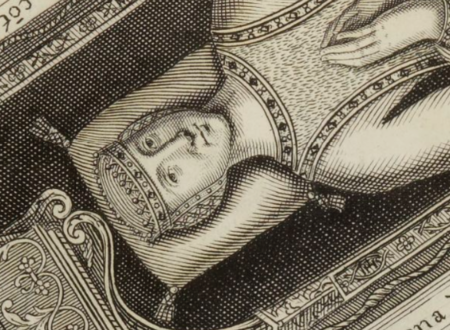
Pour les articles homonymes, voir Jeanne d'Auvergne et Jeanne Ire. Jeanne Ire d'Auvergne et de Boulogne Jeanne d'Auvergne (à gauche). Miniature de Jean Fouquet dans les Grandes Chroniques de France vers 1455. Titre Reine de France 22 août 1350 – 29 septembre 1360(10 ans, 1 mois et 7 jours) Couronnement 26 septembre 1350en la cathédrale Notre-Dame de Reims Prédécesseur Blanche de Navarre Successeur Jeanne de Bourbon Duchesse de Normandie 9 février 1350 – 22 ...
