Veria
|
Read other articles:

Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. La mise en forme de cet article est à améliorer (mars 2021). La mise en forme du texte ne suit pas les recommandations de Wikipédia : il faut le « wikifier ». Dizzy Diddy (né le 1er septembre 1988) est un artiste et entrepreneur franco-angolais[1]. Dizzy DiddyBiographieNaissance 1er septembre 1988 (35 ans)LuandaNationalité AngolaFranceActivités Artiste, entrepreneurmodifier - modifier ...

Берлинская стенанем. Berliner Mauer Карта разделенного Берлина. отмечена жёлтой линией, красные точки — контрольно-пропускные пункты Расположение Берлин Принадлежность ФРГ ГДР Германия Тип Пограничная зонаРазделённый населённый пункт Координаты Годы постр...

Статьи о герметизмеГерметизмПантеон Гермес Трисмегист Тот Гермес Меркурий Германубис Агатодемон Амон Асклепий Исида Гор Главные книги Герметический корпус Поймандр Асклепий Изумрудная скрижаль Пикатрикс Учения и практики Алхимия Астрология Теургия Мантика Магия Си...

This is a list of notable people who were considered deities by themselves or others. Imperial cults and cults of personality Main articles: Imperial cult and Cult of personality Who Image When Notability Pharaohs 3150–30 BCE Egyptian pharaohs were kings of Ancient Egypt, and were considered gods by their culture. Their titles equated them with aspects of the likes of the hawk god Horus, the vulture goddess Nekhbet, and the cobra-goddess Wadjet. The Egyptians believed that when their ...

Perlindungan dari anon Rob RiggleRiggle at the 2014 San Diego Comic-Con InternationalLahirRobert Allen Riggle, Jr.[1]21 April 1970 (umur 53)Louisville, Kentucky, U.S.PekerjaanActor, comedian, U.S. MarineTahun aktif1998–presentSuami/istriTiffany Riggle (m. 2000)Anak2Karier militerPengabdianAmerika SerikatDinas/cabangUnited States Marine Corps ReservePangkatLetnan KolonelPerang/pertempuranKosovo WarWar in Afghanistan (2001–present)Penghargaa...

American legal drama This article is about the 1963 ABC TV program. For the 2000 syndicated TV program, see Arrest & Trial. Arrest and TrialChuck Connors as John Egan and guest star Joseph Schildkraut as his clientGenreCrime/legal dramaStarring Ben Gazzara Roger Perry Chuck Connors John Larch Theme music composerBronisław KaperComposerFranz WaxmanCountry of originUnited StatesOriginal languageEnglishNo. of seasons1No. of episodes30ProductionExecutive producerFrank P. RosenbergProducers A...

Kereta Api Cut Meutia ka CUT MEUTIA Kreung Geukeuh ⇋ Kutablang pp KRD-I Cut Meutia dengan livery terbaruInformasi umumJenis layananKomuter EkonomiStatusBeroperasiDaerah operasiSub Divisi Regional I.1 AcehPendahuluKereta Api Perintis AcehMulai beroperasi 1 Desember 2013; 10 tahun lalu (2013-12-01) (sebagai KA Perintis Aceh) 3 November 2016; 7 tahun lalu (2016-11-03) (sebagai KA Cut Meutia) Terakhir beroperasi1 Juli 2014; 9 tahun lalu (2014-07-01) (sebagai KA Perintis Aceh)Oper...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: PowerBook 140 – news · newspapers · books · scholar · JSTOR (April 2023) (Learn how and when to remove this template message) Laptop by Apple PowerBook 140Apple Macintosh PowerBook 140DeveloperApple ComputerProduct familyPowerBookTypeLaptopRelease date140: Octo...

Legal management of drugs and restricted substances For laws prohibiting other drugs, see Prohibition of drugs. Methylphenidate, in the form of Ritalin pills The regulation of therapeutic goods, defined as drugs and therapeutic devices, varies by jurisdiction. In some countries, such as the United States, they are regulated at the national level by a single agency. In other jurisdictions they are regulated at the state level, or at both state and national levels by various bodies, as in Austr...

Political proposal A Celtic union or Celtic alliance refers to political unity between the Celtic nations either within the UK or together as independent countries. Historical proposals In 1864, Charles De Gaulle proposed a Celtic Union that would establish and develop links between Celtic countries. There should also be a Celtic Esperanto to facilitate communication and which would be created from common elements in all Celtic languages and a Pan-Celtic festival.[1][2]:&#...

Сельское поселение России (МО 2-го уровня)Новотитаровское сельское поселение Флаг[d] Герб 45°14′09″ с. ш. 38°58′16″ в. д.HGЯO Страна Россия Субъект РФ Краснодарский край Район Динской Включает 4 населённых пункта Адм. центр Новотитаровская Глава сельского пос�...

Para otros usos de este término, véase Nuevo León (desambiguación). Nuevo León Estado De arriba a abajo, izquierda y derecha; Vista panorámica de Monterrey, Catedral de Monterrey, Edificios en San Pedro Garza García, Parroquia de Santiago Apóstol en Villa de Santiago, Grutas de Bustamante, Palacio de gobierno del estado de Nuevo León, Edificios en la zona metropolitana de Monterrey y Museo del Obispado Escudo Lema: Semper Ascendens(latín, «Siempre Ascendiendo»). Localización de ...

Alberta Bair Theater, 2024 The Alberta Bair Theater is a performing arts center in Billings, Montana. It hosts performances ranging from local groups to nationally touring performers. Its address is 2801 3rd Ave North, on land that was previously the homestead of businessman Charles M. Bair, located in what is now downtown Billings. The theater was originally named the Fox Theater, but in 1987, the theater was renamed the Alberta Bair Theater in honor of his daughter Alberta in 1987, who made...

Circle of immediate corresponding curvature of a curve at a point Kissing circles redirects here. For Descartes' theorem on mutually tangent (kissing) circles, see Descartes' theorem. An osculating circle Osculating circles of the Archimedean spiral, nested by the Tait–Kneser theorem. The spiral itself is not drawn: we see it as the locus of points where the circles are especially close to each other.[1] An osculating circle is a circle that best approximates the curvature of a curv...

American sports company This article contains content that is written like an advertisement. Please help improve it by removing promotional content and inappropriate external links, and by adding encyclopedic content written from a neutral point of view. (November 2021) (Learn how and when to remove this message) Fenway Sports GroupCompany typePrivateIndustrySports investmentFoundedDelaware (2001 (2001))FounderJohn W. HenryTom WernerHeadquartersBoston, Massachusetts, United StatesKey peo...

For other uses, see Sandhurst (disambiguation). Human settlement in EnglandSandhurstDaffodills in Ambarrow CourtSandhurstLocation within BerkshirePopulation20,383 (2021 Census)OS grid referenceSU836618Civil parishSandhurst[1]DistrictBracknell ForestShire countyBerkshireRegionSouth EastCountryEnglandSovereign stateUnited KingdomPost townSANDHURSTPostcode districtGU47Dialling code013440127601252PoliceThames ValleyFireRoyal BerkshireAmbulanceSouth Centr...

壹環One WanChai壹環 (攝於2013年12月)概要用途住宅、商鋪地址香港灣仔灣仔道1號坐标22°16′29″N 114°10′27″E / 22.27466°N 114.17426°E / 22.27466; 114.17426前身舊灣仔街市竣工日2013年首季(11年樓齡)技术细节座数1层数35设计与建造开发商華人置業其他信息设施會所、園藝花園、停車場网站壹環 官方網站地圖 壹環前身為舊灣仔街市 住宅入口 壹環(英語:One WanChai)�...
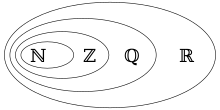
Number representing a continuous quantity For the real numbers used in descriptive set theory, see Baire space (set theory). In mathematics, a real number is a number that can be used to measure a continuous one-dimensional quantity such as a distance, duration or temperature. Here, continuous means that pairs of values can have arbitrarily small differences.[a] Every real number can be almost uniquely represented by an infinite decimal expansion.[b][1] The real number...

Questa voce sull'argomento stagioni delle società calcistiche italiane è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Voce principale: Associazione Calcio Ancona. Unione Sportiva Anconitana-BianchiStagione 1938-1939Sport calcio Squadra Anconitana-Bianchi Allenatore Ferenc Hirzer Presidente Adriano Archibugi Serie B12º posto. Maggiori presenzeCampionato: Finotto, Pincelli (33) Miglior marcatoreC...

Massacres in Transylvania The 1848–1849 massacres in Transylvania were committed in the Hungarian Revolution of 1848. According to Hungarian historian Ákos Egyed, 14,000 to 15,000 people were massacred in Transylvania in this period. The victims comprised 7,500–8,5000 Hungarians, 4,400–6,000 Romanians, and about 500 Transylvanian Saxons, Armenians, Jews, and members of other groups.[1] Massacres of Hungarians On 18 October 1848, Romanians attacked and murdered inhabitants of th...
